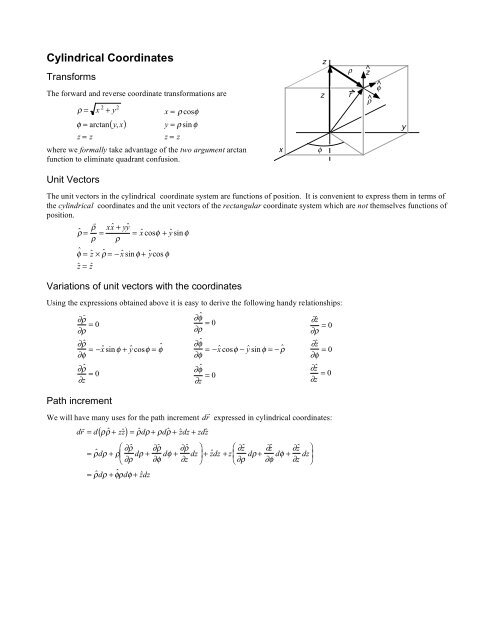
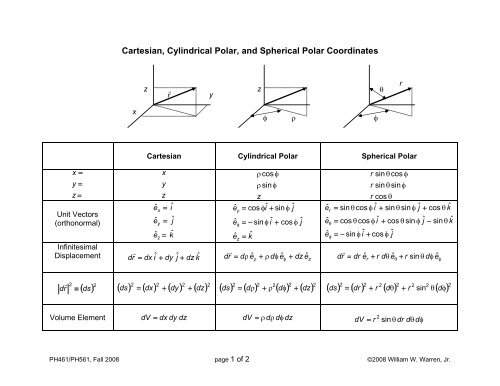
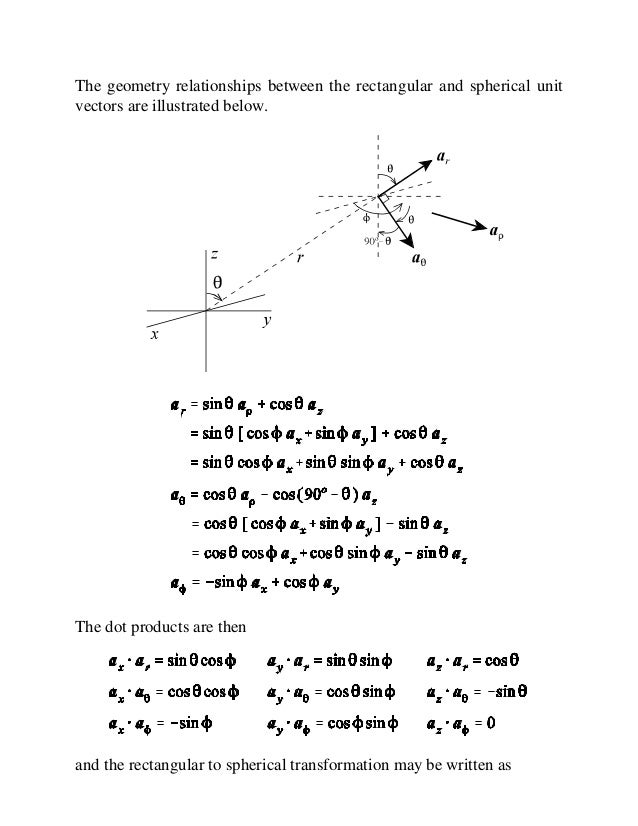
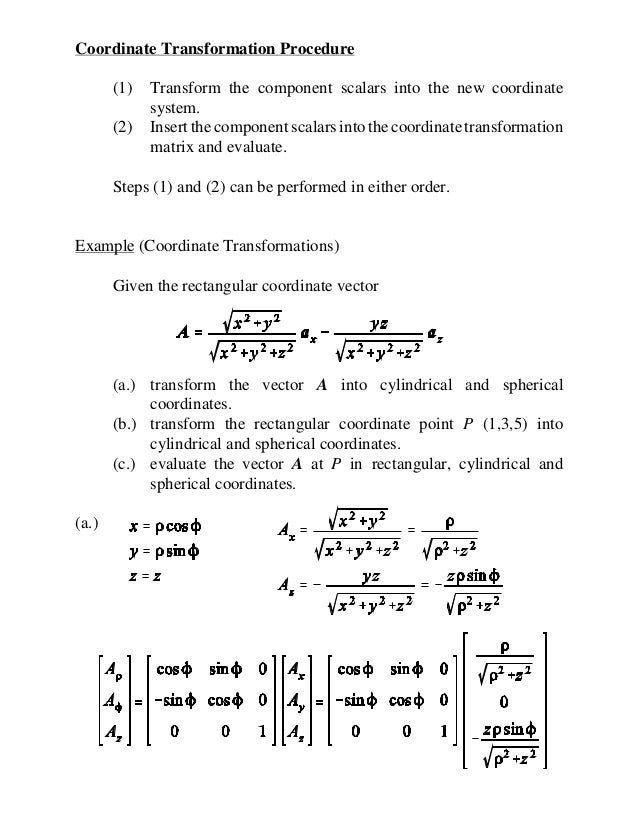
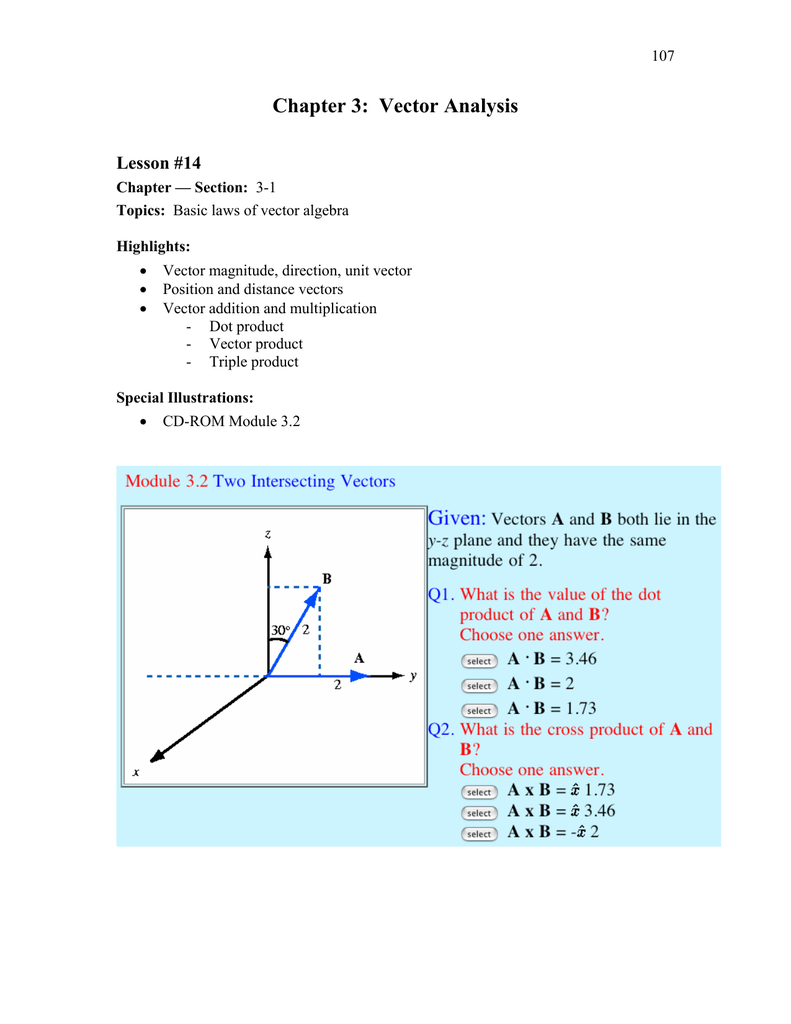
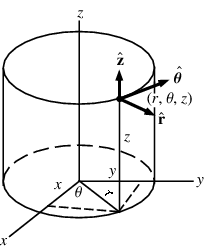
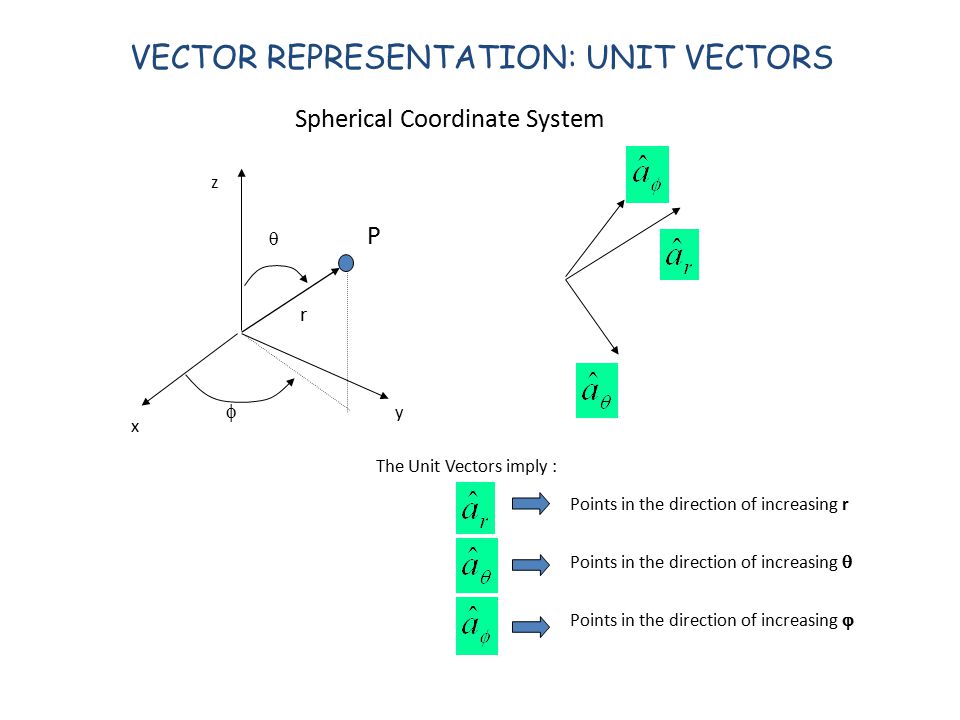
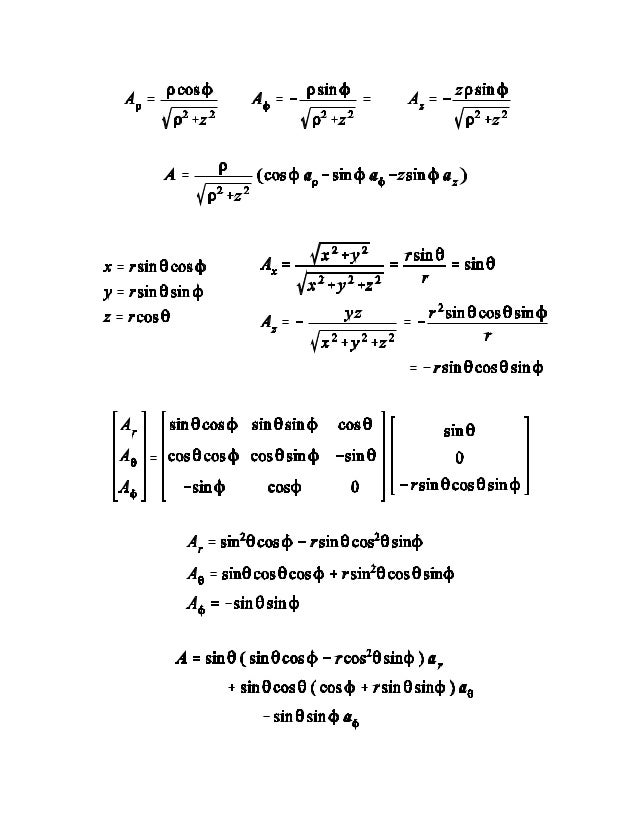
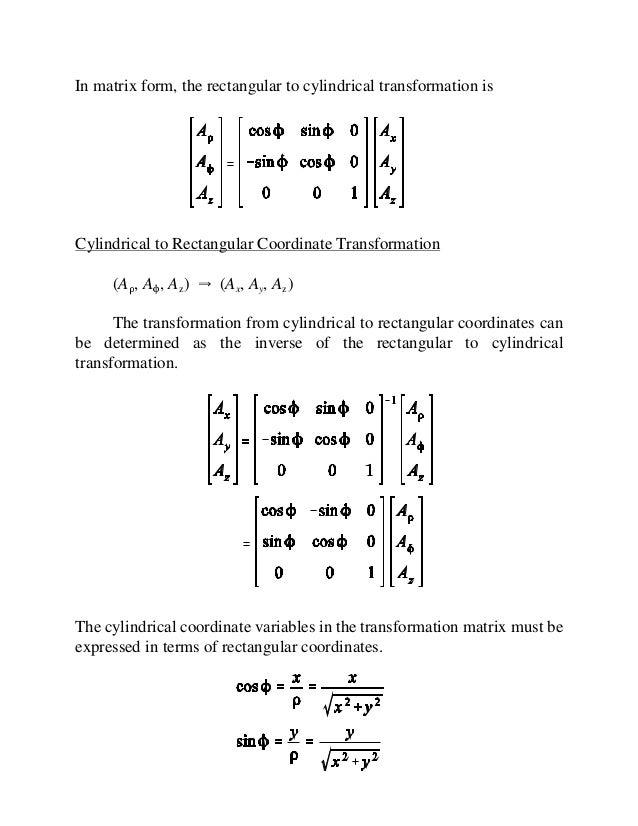
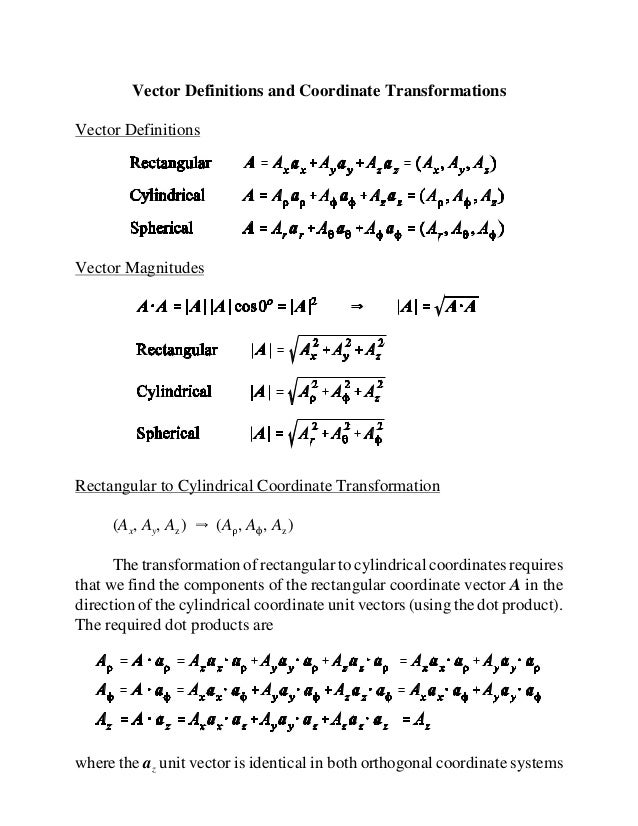
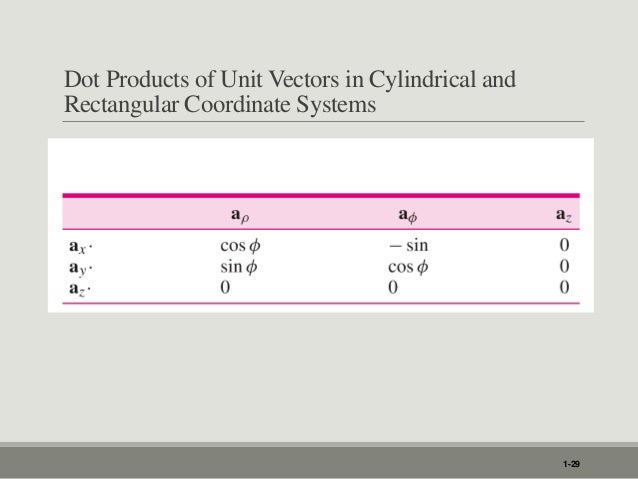
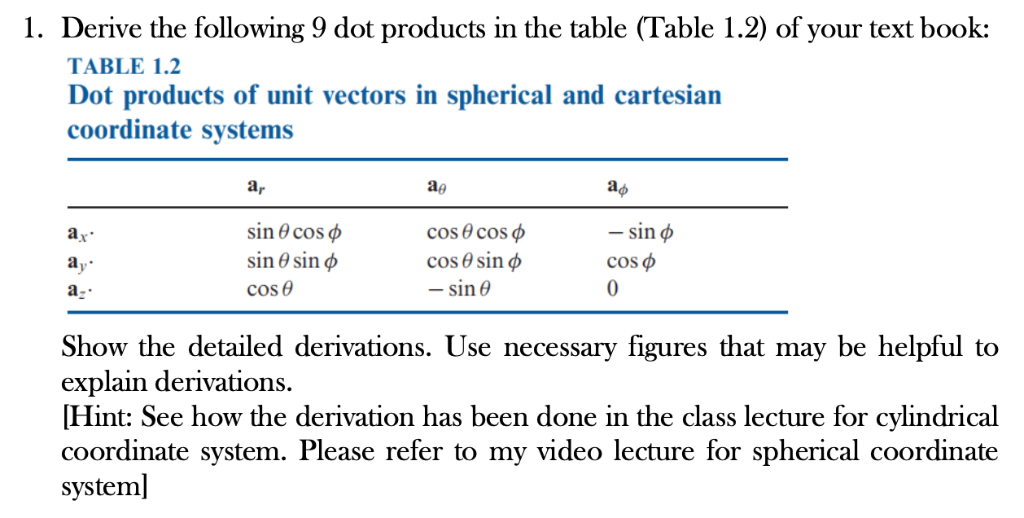
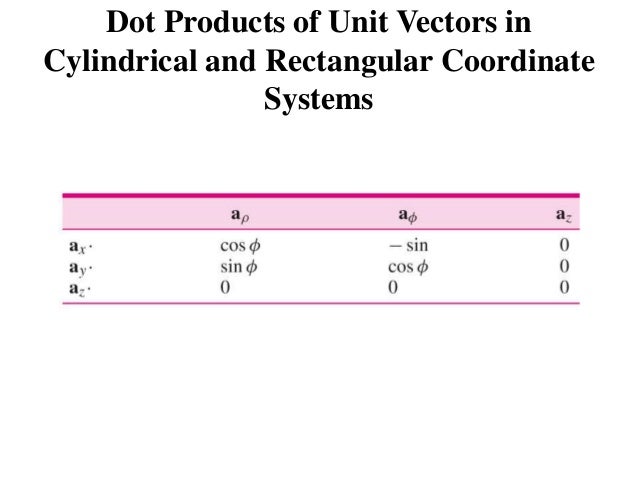
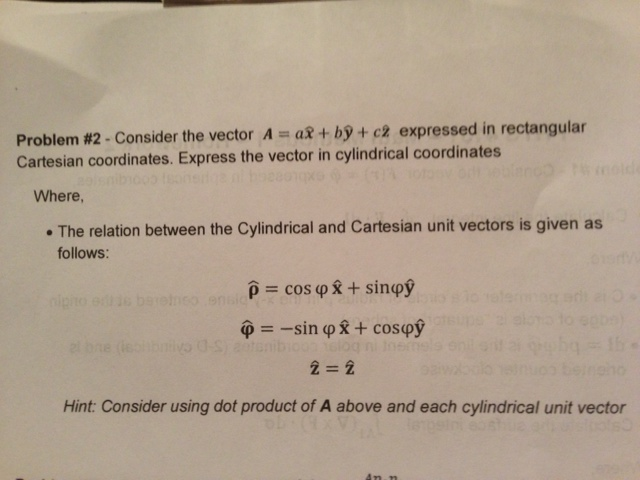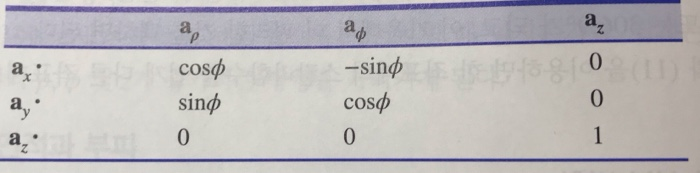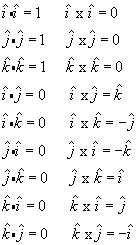Dot Product Of Unit Vectors In Cylindrical Coordinates

25 of 55 cylindrical coordinatespoint and unit vectors duration.

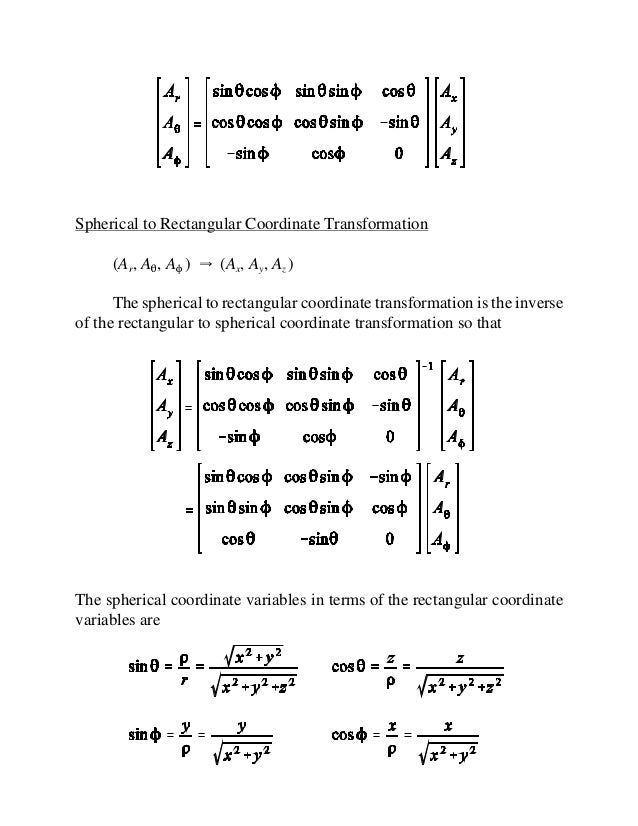
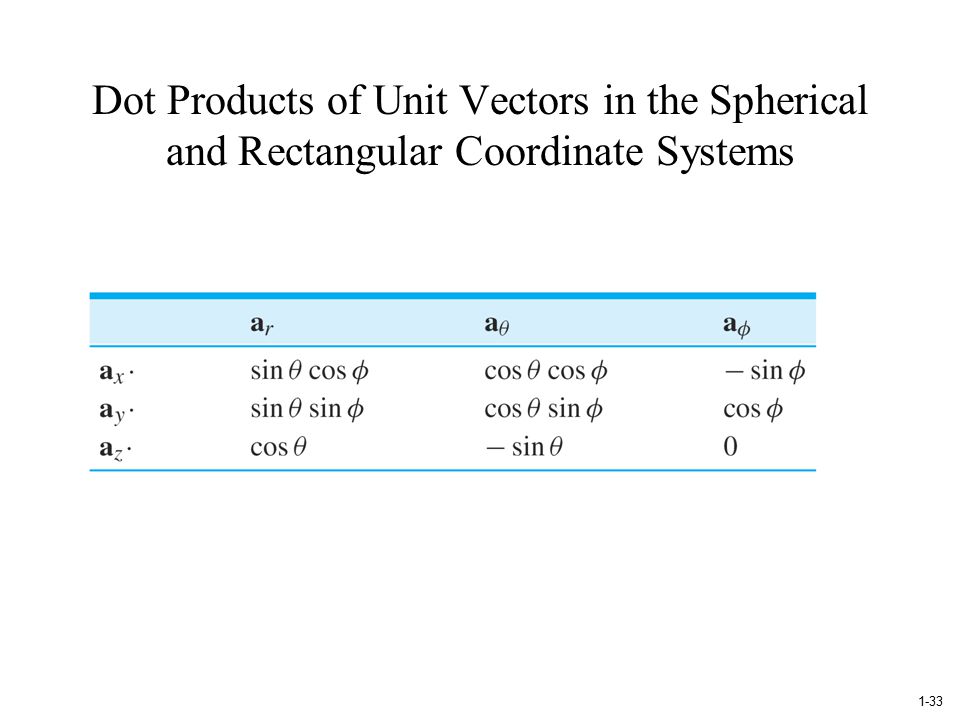
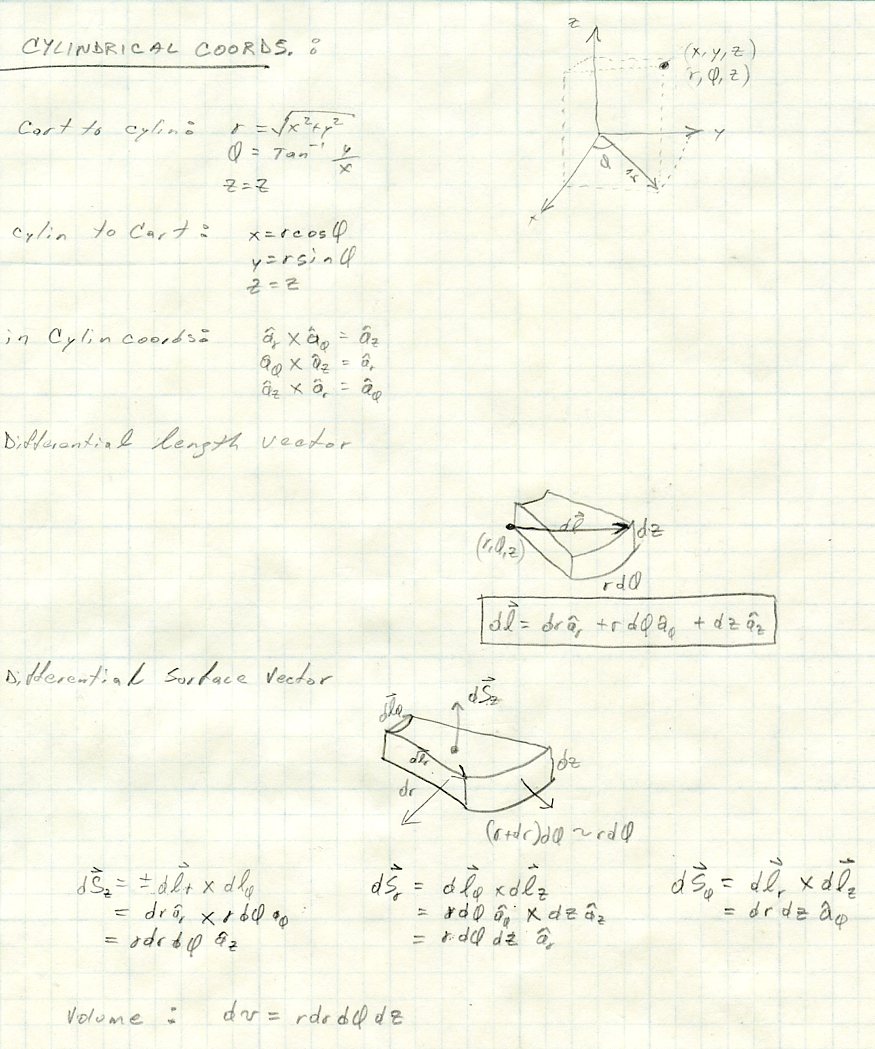
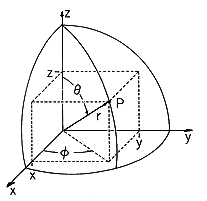
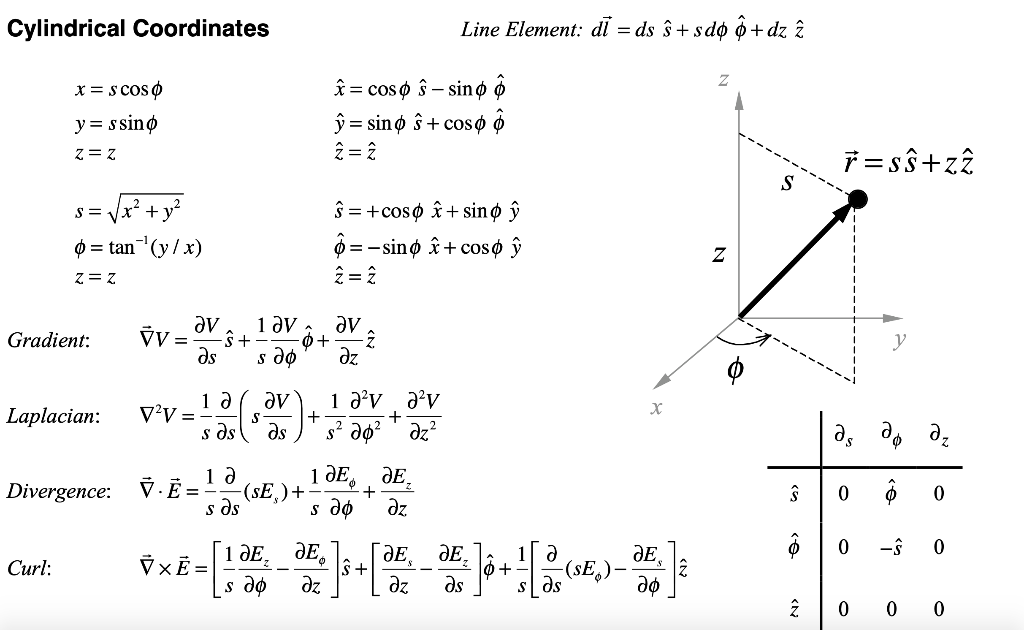
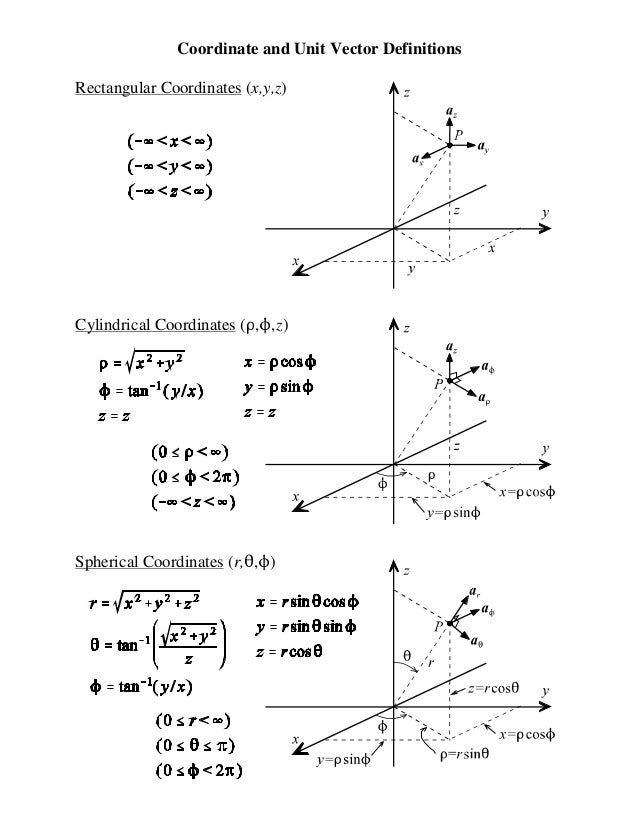
Dot product of unit vectors in cylindrical coordinates. Cylindrical coordinates are a generalization of two dimensional polar coordinates to three dimensions by superposing a height z axis. Unfortunately there are a number of different notations used for the other two coordinates. Vectors are defined in cylindrical coordinates by r f z where. You can verify this directly.

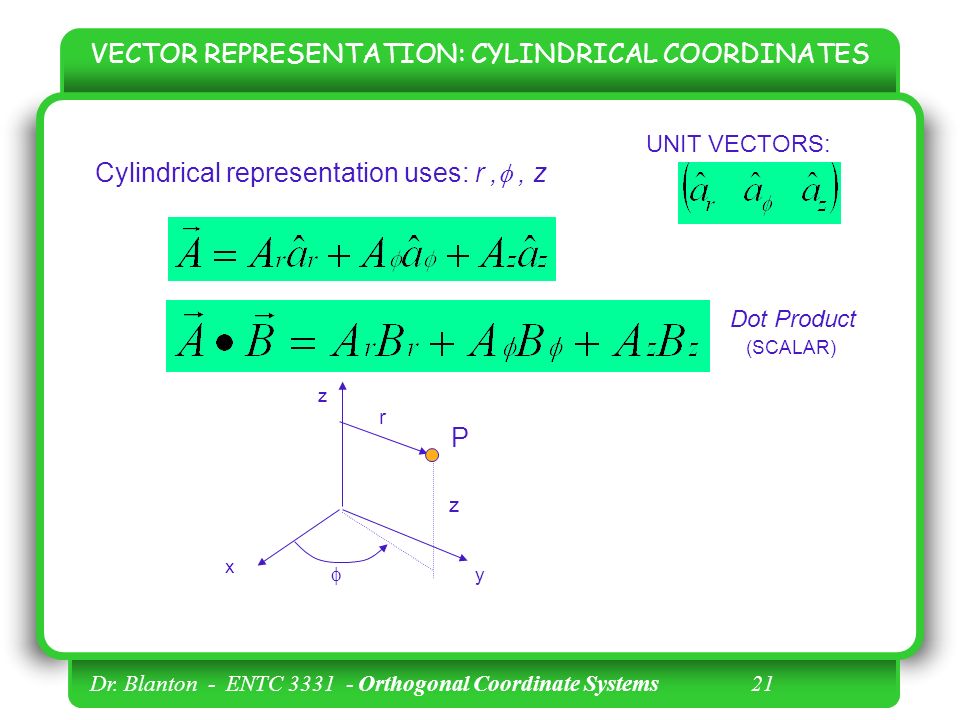
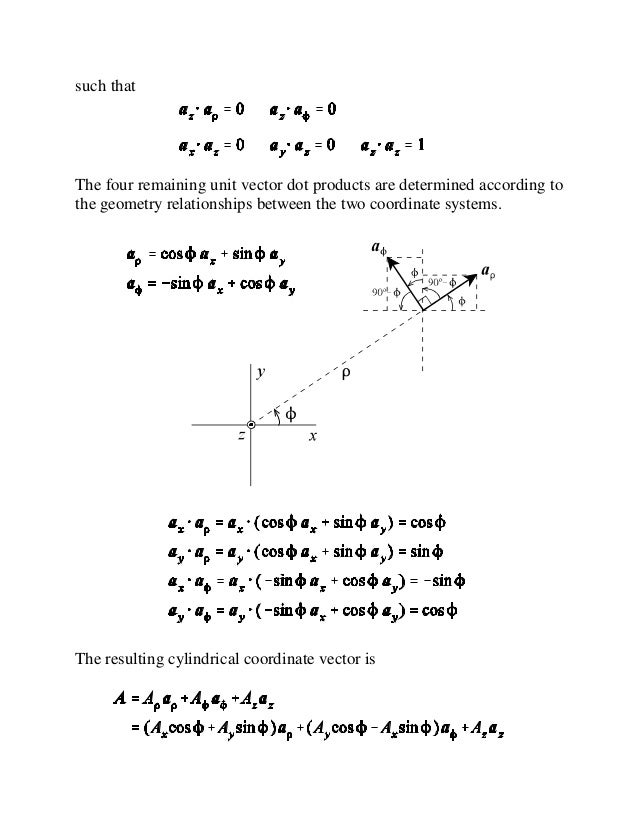
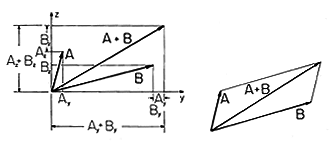
F is the angle between the projection of the vector onto the xy plane ie. It should be easy to see that these unit vectors are pairwise orthogonal so in cylindrical coordinates the inner product of two vectors is the dot product of the coordinates just as it is in the standard basis. R and the positive x axis 0 f 2p. Find the vector in cylindrical coordinates a ar ar af af a z a z to find any desired component of a vector we take the dot product of the vector and a unit vector in the desired direction.

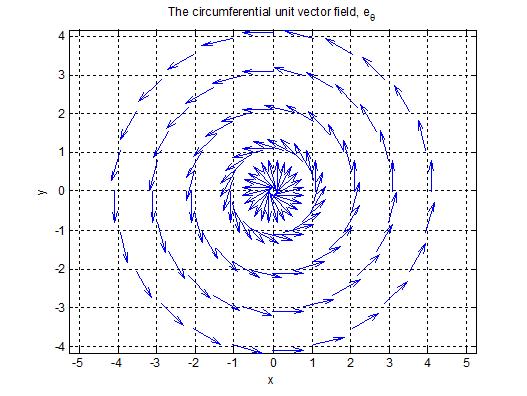
Cylindrical coordinate system vector fields. Ar a ar and a f a af. R is the length of the vector projected onto the xy plane. With a little bit of work we can find that so that and similarly for.

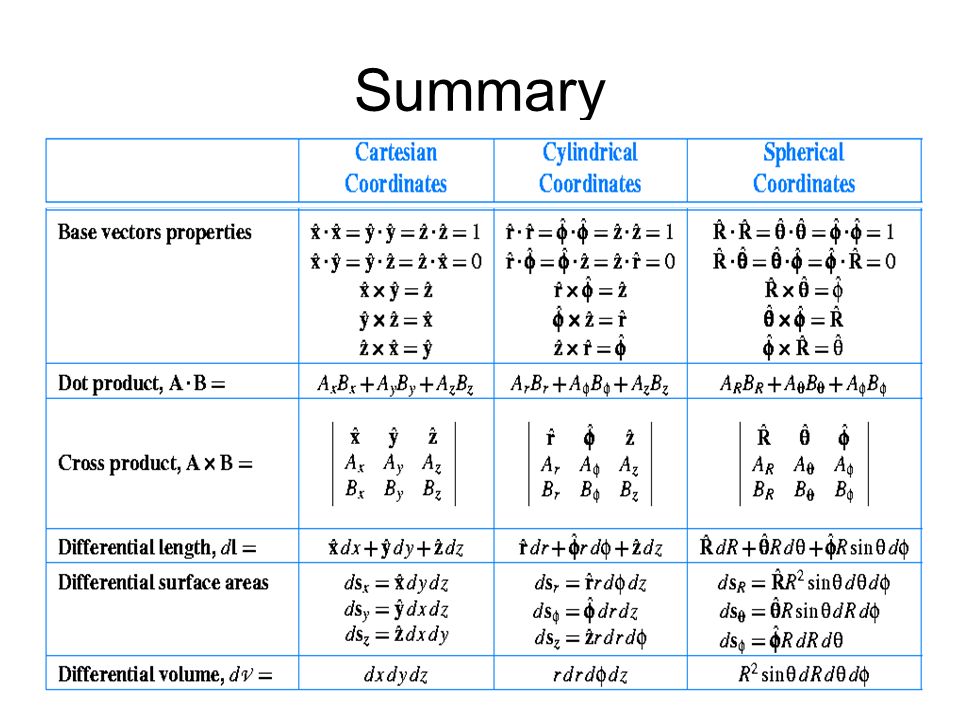
23 circular cylindrical coordinates2 r9 f z finding dot or cross product of two vectors in a cylindrical system is the same as that used in the cartesian system in chapter 1. R f z is given in cartesian coordinates by. Trick to remember formula of dot product of cordinate system. Michel van biezen 68914 views.