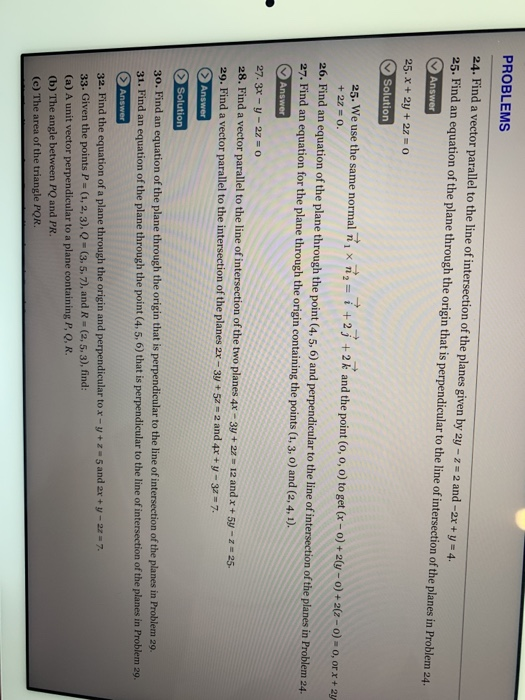
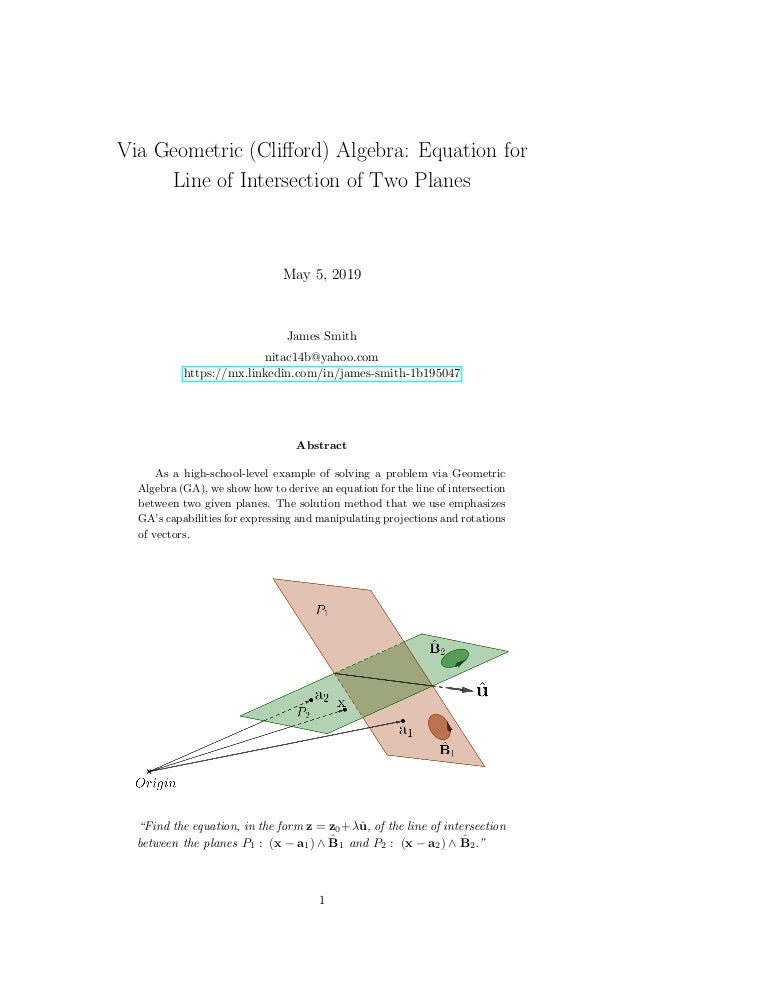
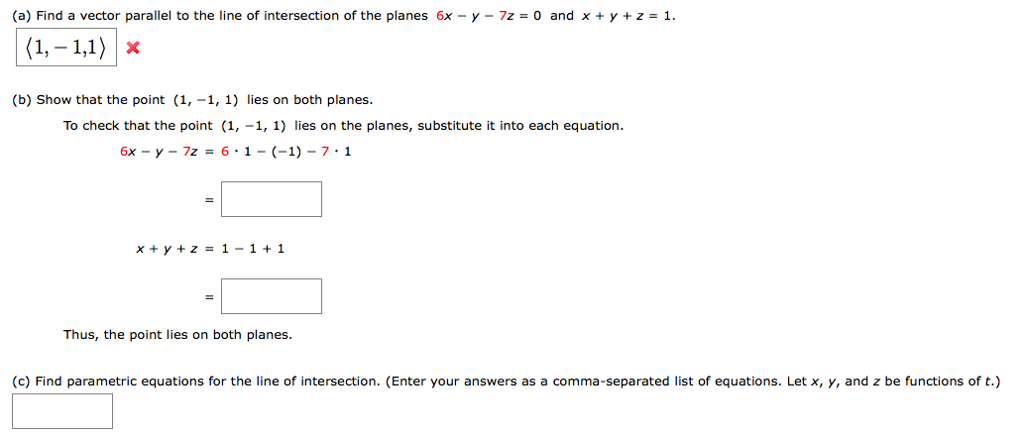
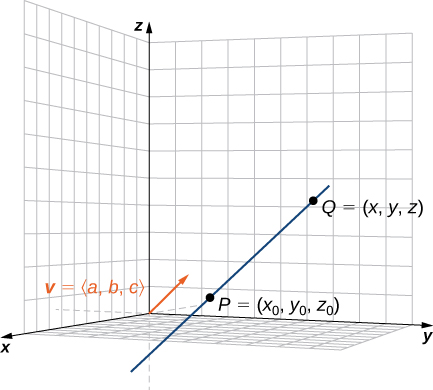
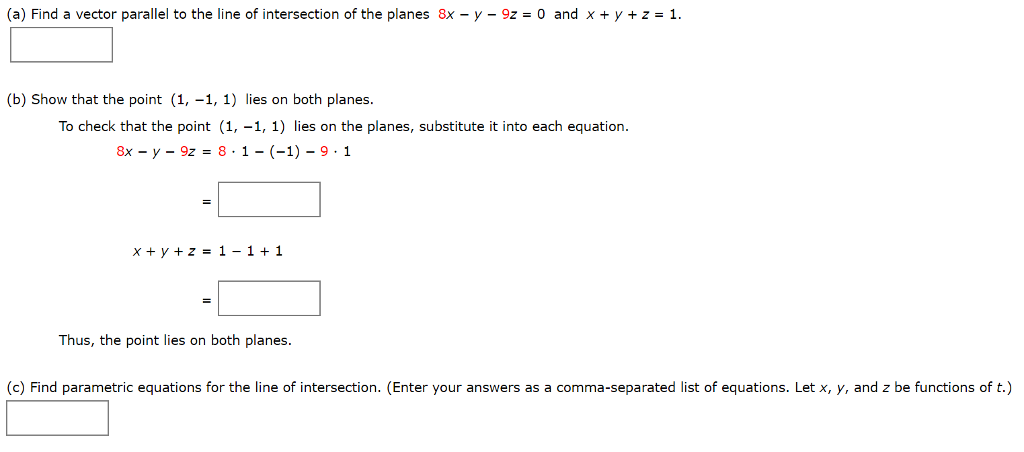
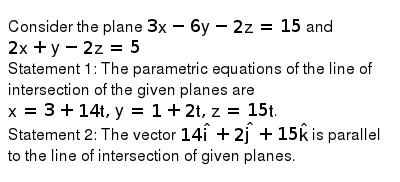
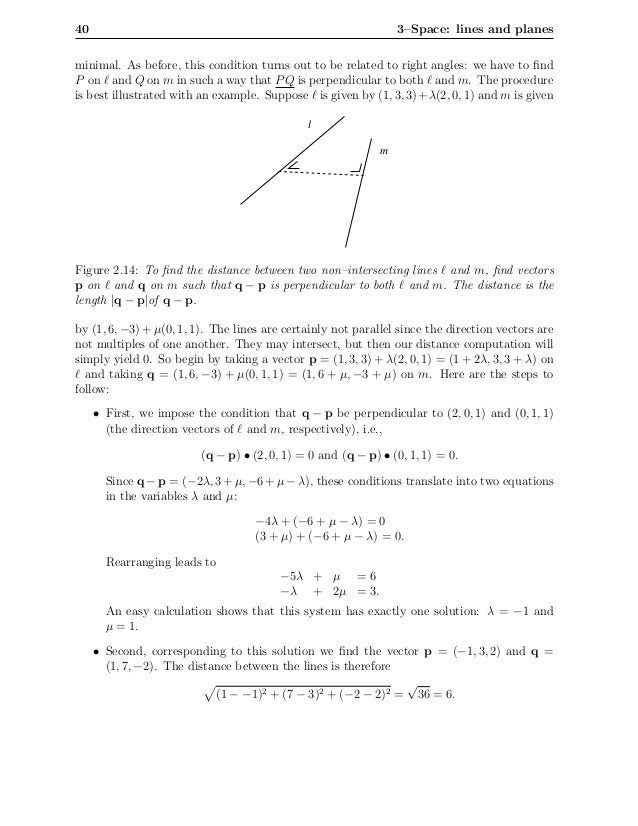
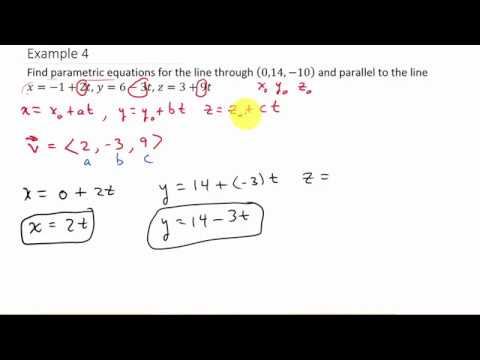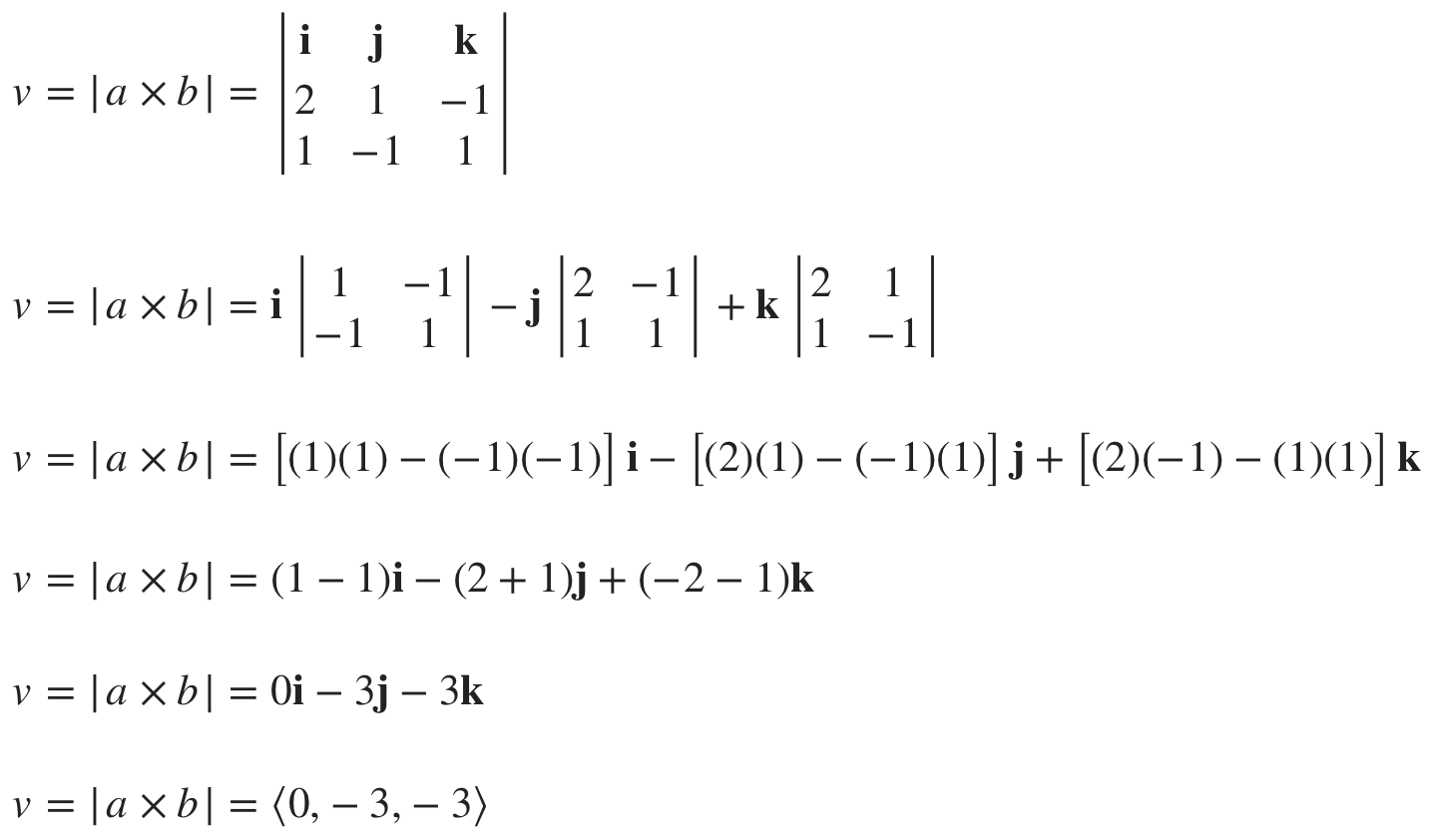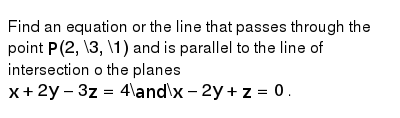Find A Vector Parallel To The Line Of Intersection Of The Planes

That you can do by setting one of the variables to 0 and solving it. L 1 3i j 2k l 2 2i j 4k. Find a vector parallel to the line of intersection of the planes given by the equations 2x 3y 5z 2 and 4x y 3z 7.
find a vector parallel to the line of intersection of the planes find a vector parallel to the line of intersection of the planes calculator


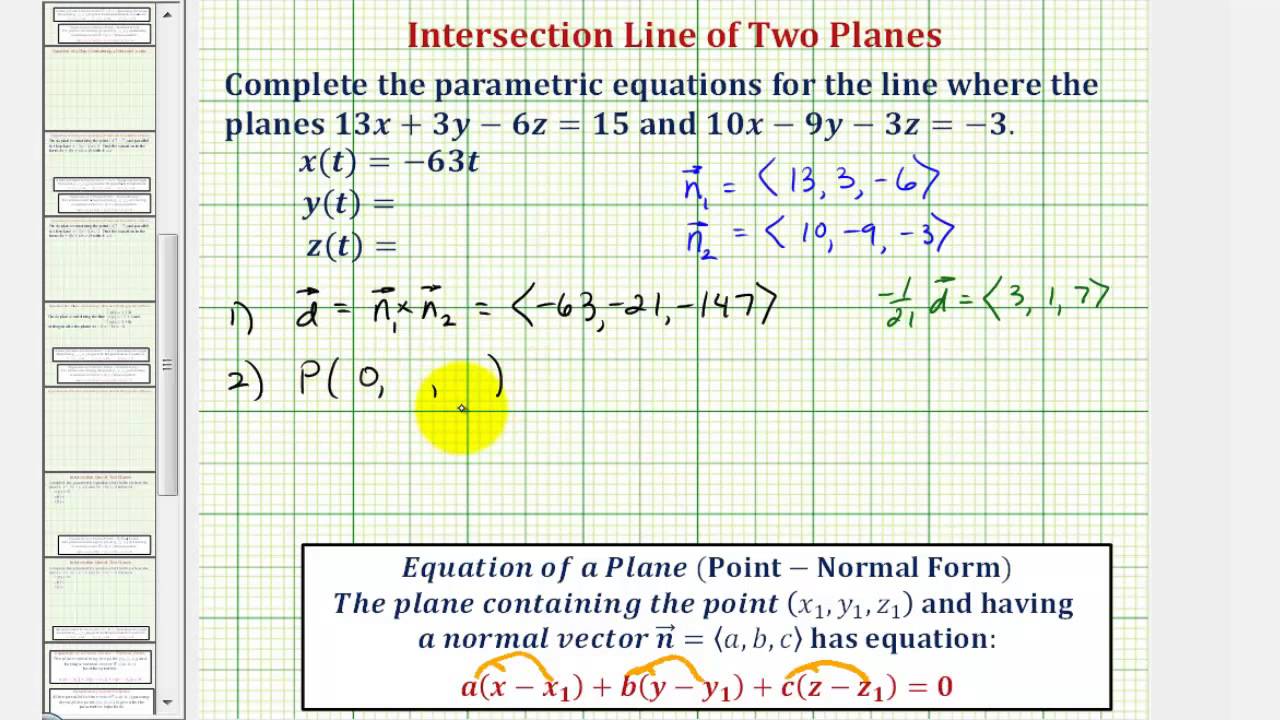
Then the equation of the line is 5 2 11 3 1 6 t a.

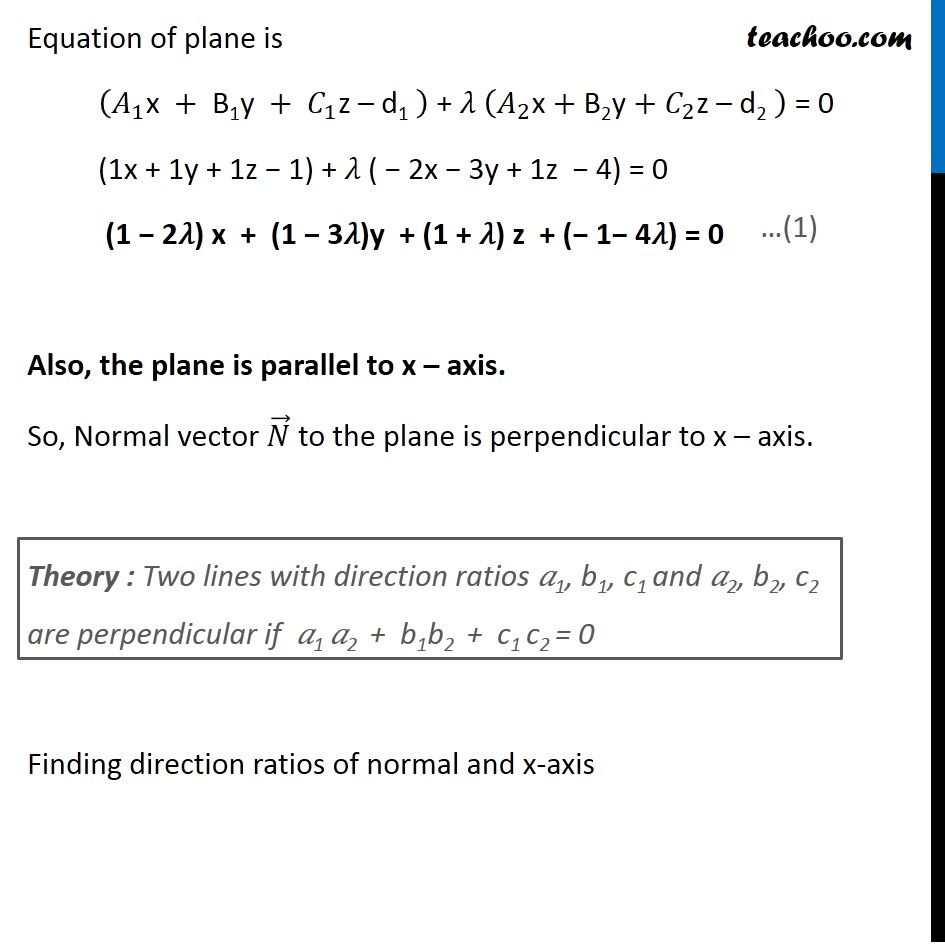
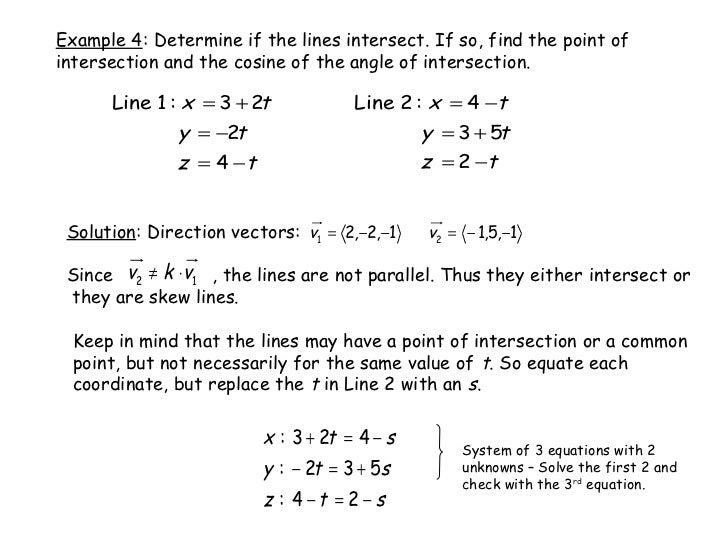
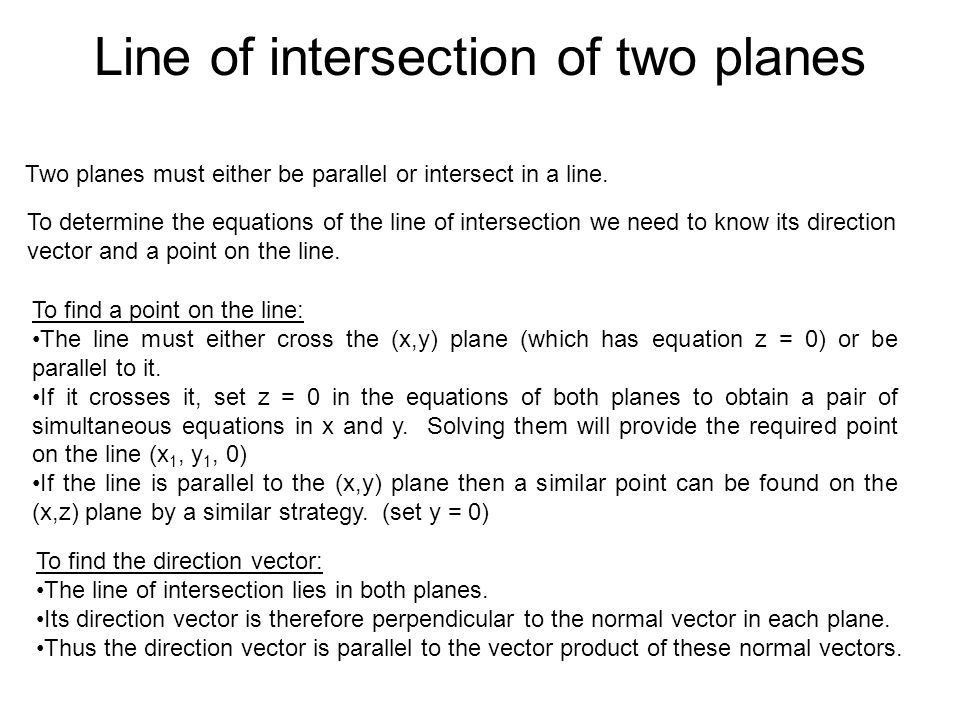
Find a vector parallel to the line of intersection of the planes. The general vector direction of the perpendicular lines to the first and second planes are the coefficients x y and z of the planes equations. Adding the two equations i choose the two points of intersection of the planes to be p 3 1 6 and x 5 2 11. Line of intersection of the two planes is perpendicular to both vectors. If not find the equation of the line of intersection in parametric and symmetric form.

So the vector t a 2 1 5. Then you find vector parallel to the line. Next we nd the direction vector d for the line of intersection by computing d n. To find direction vector of this line simply take the cross product of the two vectors above.

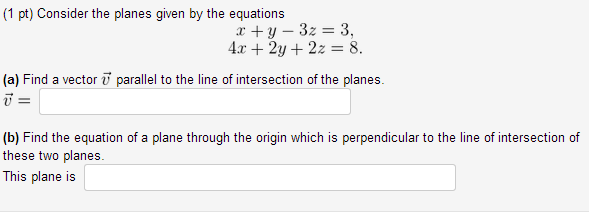
Simply you find a point where the line of intersection intersects with one of the planes x y y z x z it must with at least one of them. The given planes are 2 x y z 1 and 3 x y z 2. First we read o the normal vectors of the planes. A find a vector parallel to the line of intersection of the planes 5x 2y 2z 5 and 2x 2y 4z 0.

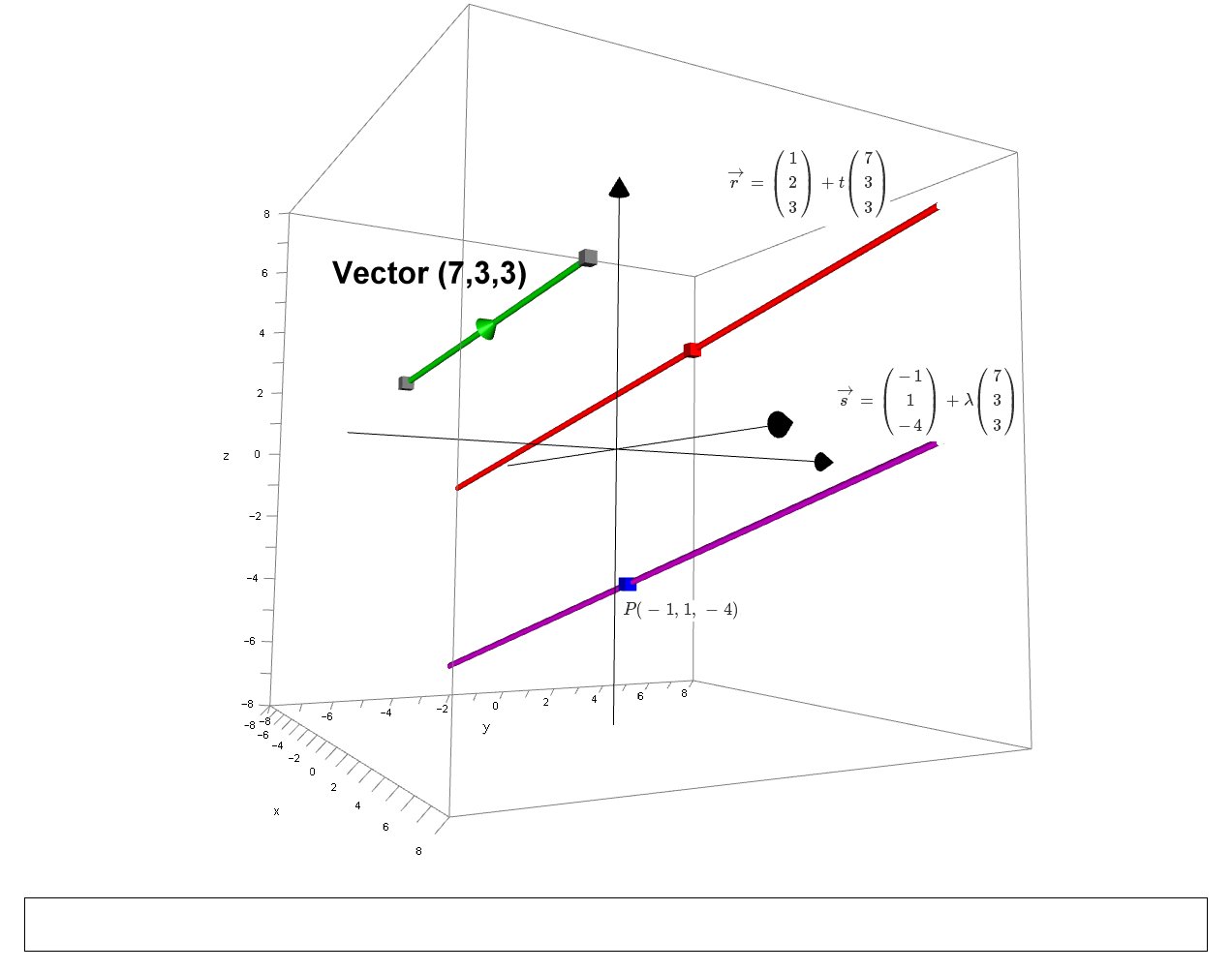
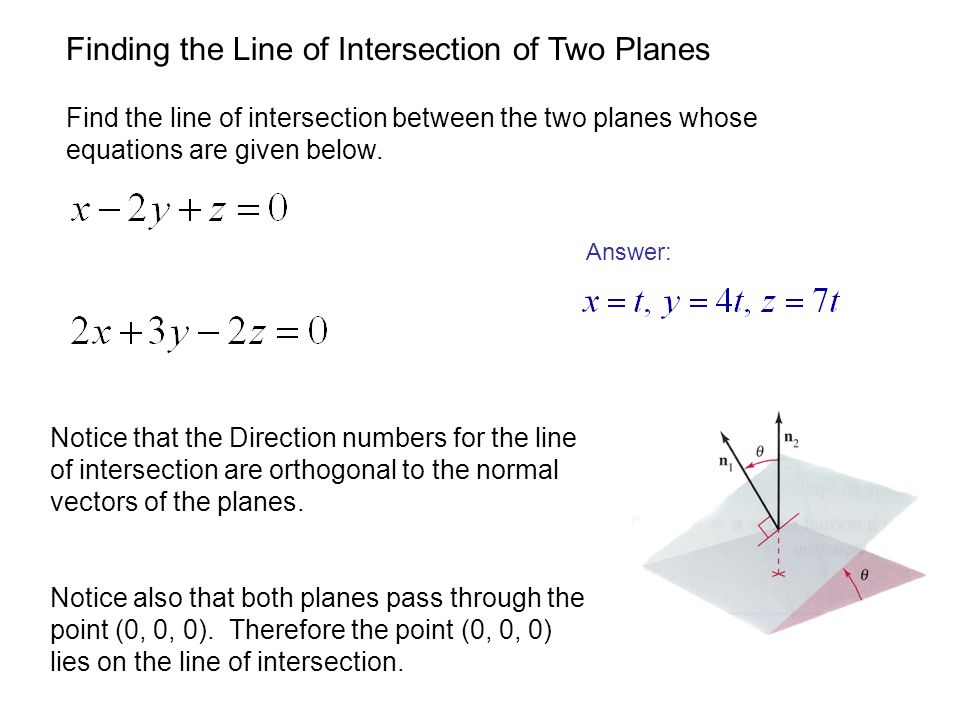
The intersection line can also be found by vector method. Find a vector equation of the line of intersections of the two planes x 1 5x 2 3x 3 11 and 3x 1 2x 2 2x 3 7. 4 3 5 x 2 4 1. The normal vector n 1 of x 1 5x 2 3x 3 11 is 2 4 1 5 3 3 5 and the normal vector n 2 of 3x 1 2x 2 2x 3 7 is 2 4 3 2 2 3 5.

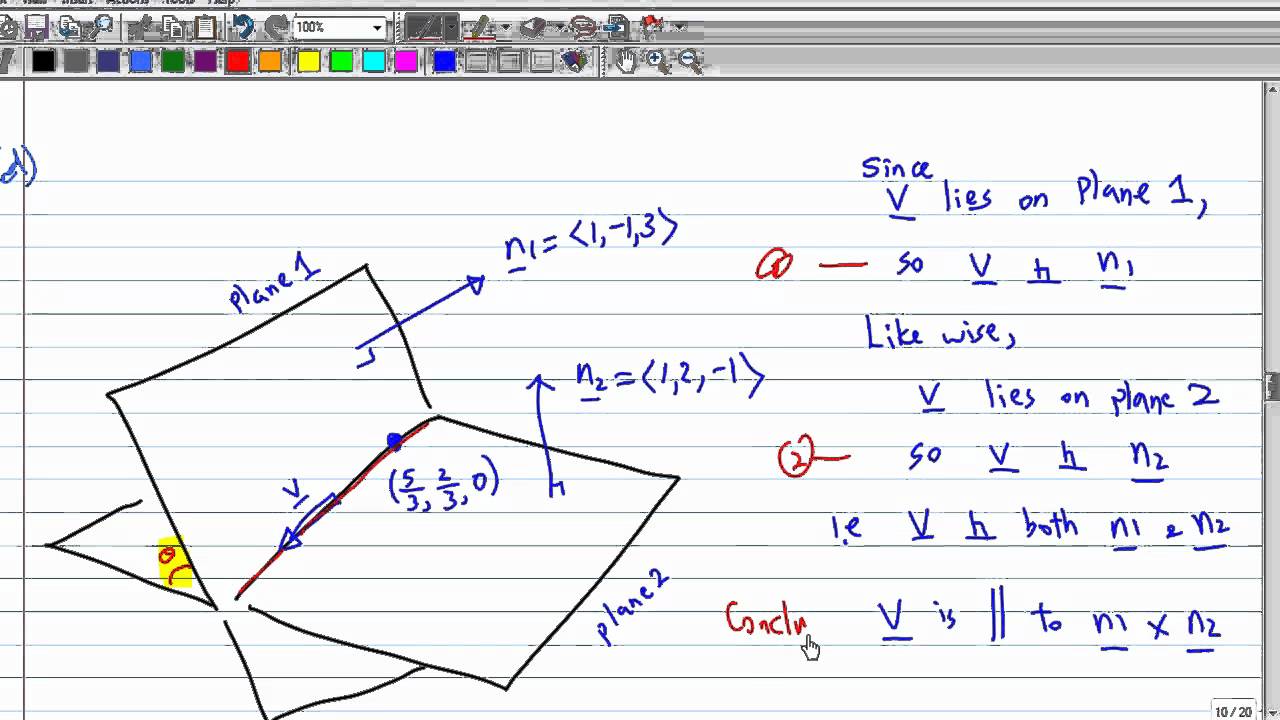
Then find a vector parametric equation for the line of intersection. V b show that the point 1 1 1 lies on both planes. Are the planes 2x 3y z 4 and x y z 1 parallel. The cross product is used.

3 3 1 x 3 2 2 7 6 3. Favorite answer the cross product of the normal vectors of the two planes will be parallel to the line of intersection.

Misc 15 Plane Intersection Of Planes Parallel To X Axis

Misc 15 Plane Intersection Of Planes Parallel To X Axis

The Line Of Intersection Of The Planes X 2y 3z 1 And X Y

The Line Of Intersection Of The Planes X 2y 3z 1 And X Y

Line Of Intersection Of Two Planes Youtube

Line Of Intersection Of Two Planes Youtube

How To Find The Vector Equation Of The Line Of The Intersection Of

How To Find The Vector Equation Of The Line Of The Intersection Of

Prove That The Line Of Intersection Of Two Non Parallel Planes Is

Prove That The Line Of Intersection Of Two Non Parallel Planes Is

Solved Q1 Find A Vector Parallel To The Line Whose Carte
Equations Of Planes
Find The Equation Of The Plane Through The Line Of Intersection Of
Consider The Line Which Passes Through The Point P 1 1 4 And
Equations Of Lines And Planes
Https Encrypted Tbn0 Gstatic Com Images Q Tbn 3aand9gcr7dqbptb14lrikox6cx1xzkthdjpkvnquslk Lwd L6kqjgt72 Usqp Cau
Misc 19 Find Vector Equation Of Line Passing Through 1 2 3 And
12 5 Equations Of Lines And Planes Mathematics Libretexts
Solved 1 Pt A Find A Vector Parallel To The Line Of I
Find Equation Of Plane Perpendicular To Two Given Planes Through
The Line Of Intersection Between Two Planes
Vector Equations
Parametric Equations For The Line Of Intersection Of Two Planes
Line Of Intersection Of Two Planes Youtube
Solved 1 Point Find A Nonzero Vector Parallel To The Li
Line Of Intersection Of Two Planes By Cross Product Youtube
Solved Problems 24 Find A Vector Parallel To The Line Of
A Unit Vector Parallel To The Intersection Of The Planes Vec
Solved Roblems 20 Find A Vector Parallel To The Line Of
Find A Vector Parallel To The Intersection Of The Planes 2x 3y 5z
7 5 Lines And Planes In Space
Solved 5 2a Find A Vector Parallel To The Line Of Int
Parallel Perpendicular And Angle Between Planes Kristakingmath
The Intersection Of Two Line Segments The Do Loop
Show That The Line Of Intersection Of The Planes X 2y 3z 8 And
Consider The Planes 2x Y Z 1 And 2x Z 0 A Find The
Vector Equations
Intersections Of Lines Segments And Planes 2d 3d
Solved Consider The Planes Given By The Equations X Y
Parallel Lines Skew Lines And Planes Solutions Examples Videos
Show That The Line Of Intersection Of The Planes X 2y 3z 8 And
How To Find The Vector Equation For The Plane That Is Parallel To
Planes Intersection Calculator
Equations Of Lines And Planes
Https Encrypted Tbn0 Gstatic Com Images Q Tbn 3aand9gctnkhjsrz1ooeyaydw59gwckaozfuyurmliobxz4wxq50jorogu Usqp Cau
Vectors Applications Nhaa Imk Unimap Ppt Download
Intersections Of Three Planes Part 1 Youtube
Determine If Lines Are Parallel Intersecting Or Skew Youtube
The Line Of Intersection Of The Planes X 2y 3z 1 And X Y
2
Analytical And Vector Geometry Docsity
Vector Equations
Finding Parametric Equations Through A Point And Parallel To A
Https Users Math Msu Edu Users Gnagy Teaching 10 Fall Mth234 W3 234 H Pdf
Line Line Intersection Wikipedia
Solved 1 Point Find A Nonzero Vector Parallel To The Li
Solved 1 Consider The Planes Given By The Equations Fi
Vector Equations
How To Find Intersection Point Of A Line In A Plane In 3d Space
12 5 Lines And Planes
Vector Line And Plane Equation A Level Maths Uptuition With Mr
Mathscene Vectors Lesson 5
Find Vector Equation Of A Line Parrallel To Given Line Passing
Https Encrypted Tbn0 Gstatic Com Images Q Tbn 3aand9gcr0hnumhrto9j4t L Yqfxs4hqawsa7wizgj1xqbe6ilsf7 Hrg Usqp Cau
Let Be Vector Parallel To The Line Of Intersection Of Planes P1
Find The Equation Of The Plane That Passes Through The Line Of
A Non Zero Vector A Is Parallel To The Line Of Toppr Com
Symmetric Equations For The Line Of Intersection Of Two Planes
Line Geometry Wikipedia
The Line Of Intersection Of Two Planes Projection Of A Line Onto
Equations Of Lines And Planes In Space Calculus Volume 3
Intersections Of Lines Segments And Planes 2d 3d
Intersections Of Lines Segments And Planes 2d 3d
Emma S Final Year Project
Via Geometric Clifford Algebra Equation For Line Of Intersection O
Equations Of Planes
Line Of Intersection For Two Planes Youtube
Ex 2 Find The Parametric Equations Of The Line Of Intersection Of
Solved A Find A Vector Parallel To The Line Of Intersec
9 5 Equations Of Lines And Planes
Equations Of Lines And Planes In Space Calculus Volume 3
The Line Of Intersection Of The Planes X 2y 3z 1 And X Y
Find A Vector Of Magnitude 4 Units Which Is Parallel To The Vec
Find The Vector Equation Of The Plane Passing Through 1 2 3 And
How To Find Parametric Equations For The Line Of Intersection Of
Vectors Addition Is Commutative Vi If Vector U Is Multiplied By
Solved Find A Vector Of Magnitude 2 Parallel To The Lin
Dot Product Cross Product Applications Ppt Video Online Download
Solved 1 Consider The Planes Given By The Equations Fi
Finding The Vector Equation For A Line That Intersects Two Planes
3d Lines And Planes Ppt Video Online Download
Vector Equations
Https Encrypted Tbn0 Gstatic Com Images Q Tbn 3aand9gcrrfqfs5j9ueadgsdey7m97yk8nphdea6akpbgbvm1abtwqeujn Usqp Cau
Let P 1 2x Y Z 3 And P 2 X 2y Z 2 Be Two Planes Then Which
Equations Involving Lines And Planes Standard Equations For Lines
Solved Consider The Planes Given By The Equations X Y 3z
Ex 2 Find The Parametric Equations Of The Line Of Intersection Of
Vector Equations
Solved A Find A Vector Parallel To The Line Of Intersec
Consider The Planes 3x 6y 2z 15a N D2x Y 2z 5 Statement 1 The
2 5 Equations Of Lines And Planes In Space Calculus Volume 3
A Vector Parallel To The Line Of Intersection Of The Planes Vec
Lines And Planes In Space
Https Faculty Math Illinois Edu Nirobles Files241 Examplescalc3 Pdf
How To Find The Line Of Intersection Between Two Planes Quora
Line Line Intersection Wikipedia
Distance Between Parallel Lines Formulas Videos And Solved Examples
Https Www Math Uh Edu Bekki 2433 Su16 Notes Sn2 1 Pdf
Find A Vector Parallel To The Line Of Intersection Of The Planes

Random Posts

Dmca Contact Privacy Policy Copyright