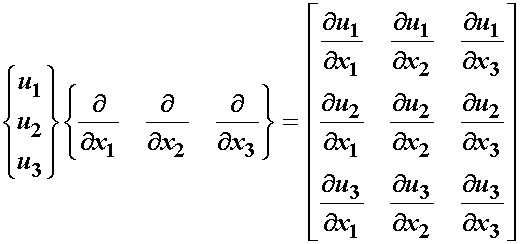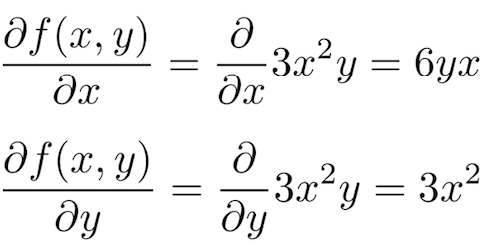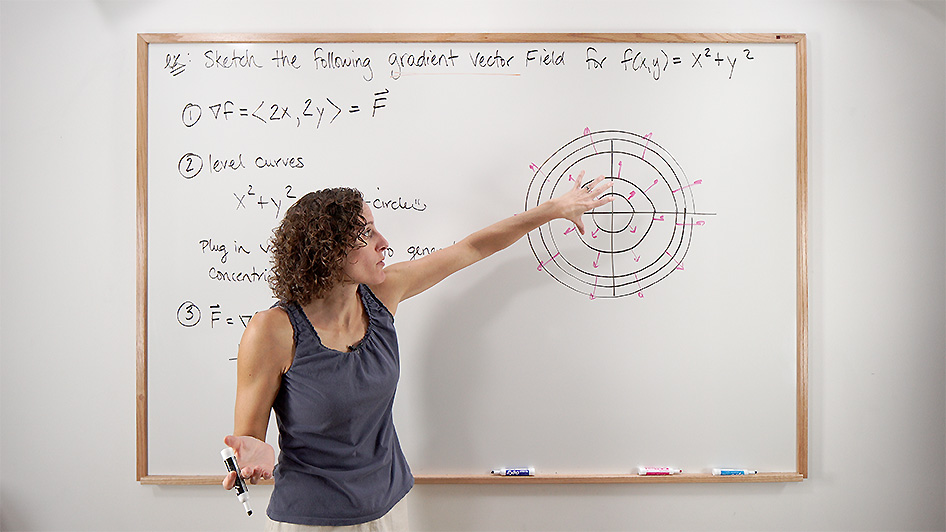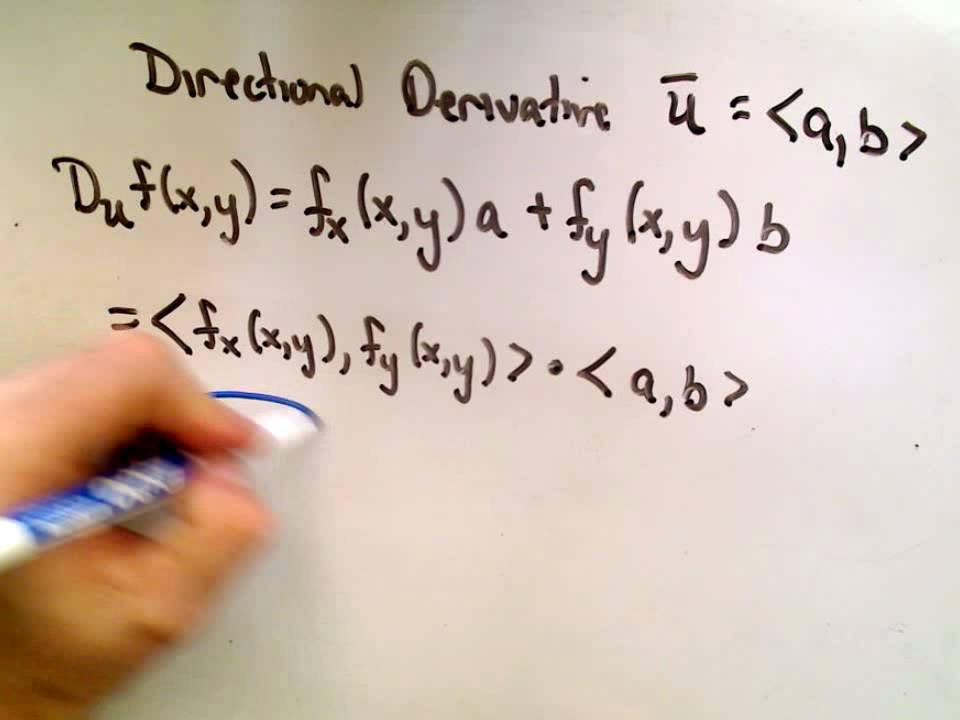Gradient Of A Vector Field Example

Given a function its gradient is.

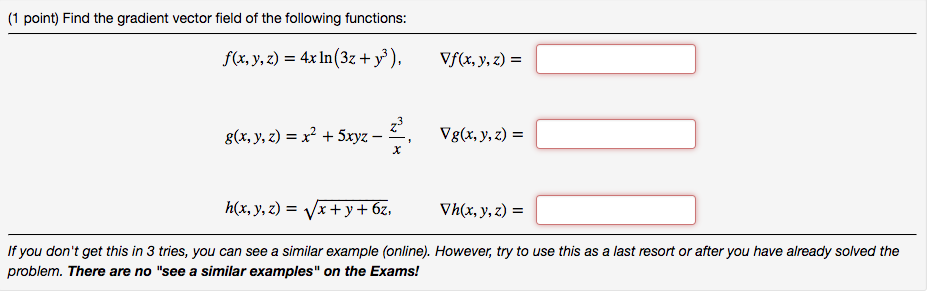
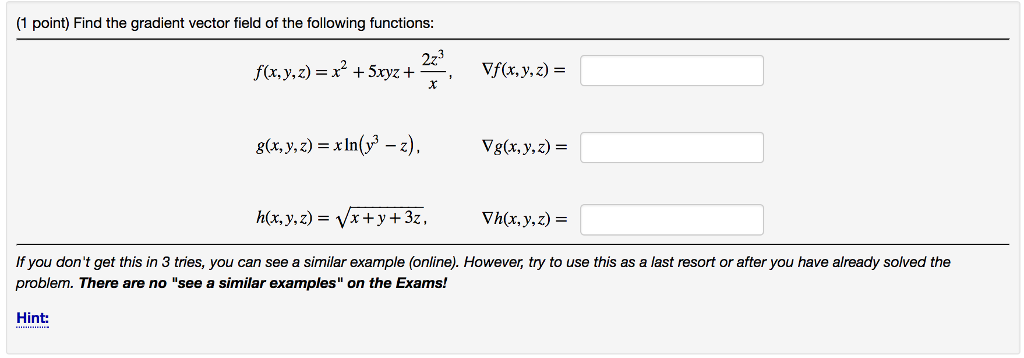
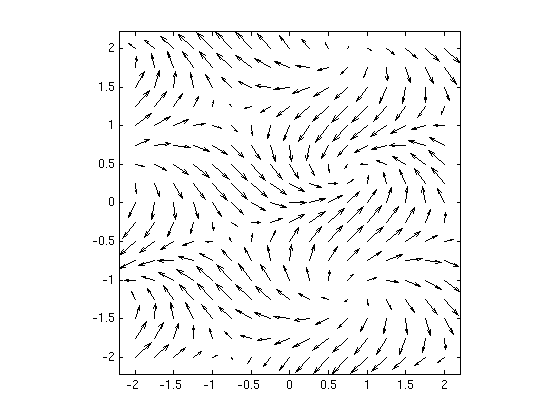
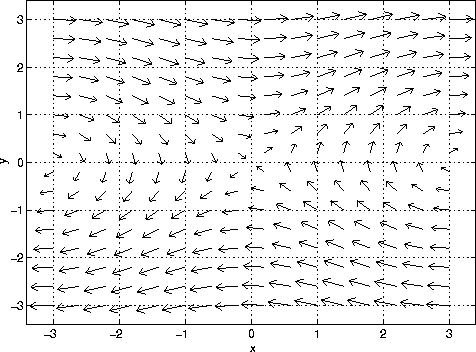
Gradient of a vector field example. F xy x2sin5y f x y x 2 sin. Its a vector a direction to move that. Example 2 find the gradient vector field of the following functions. In these cases the function f xyz f x y z is often called a scalar function to differentiate it from the vector field.

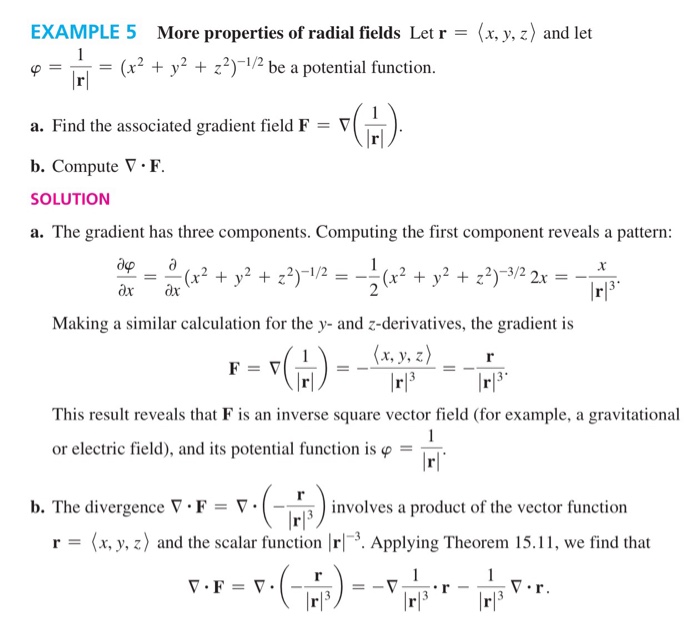
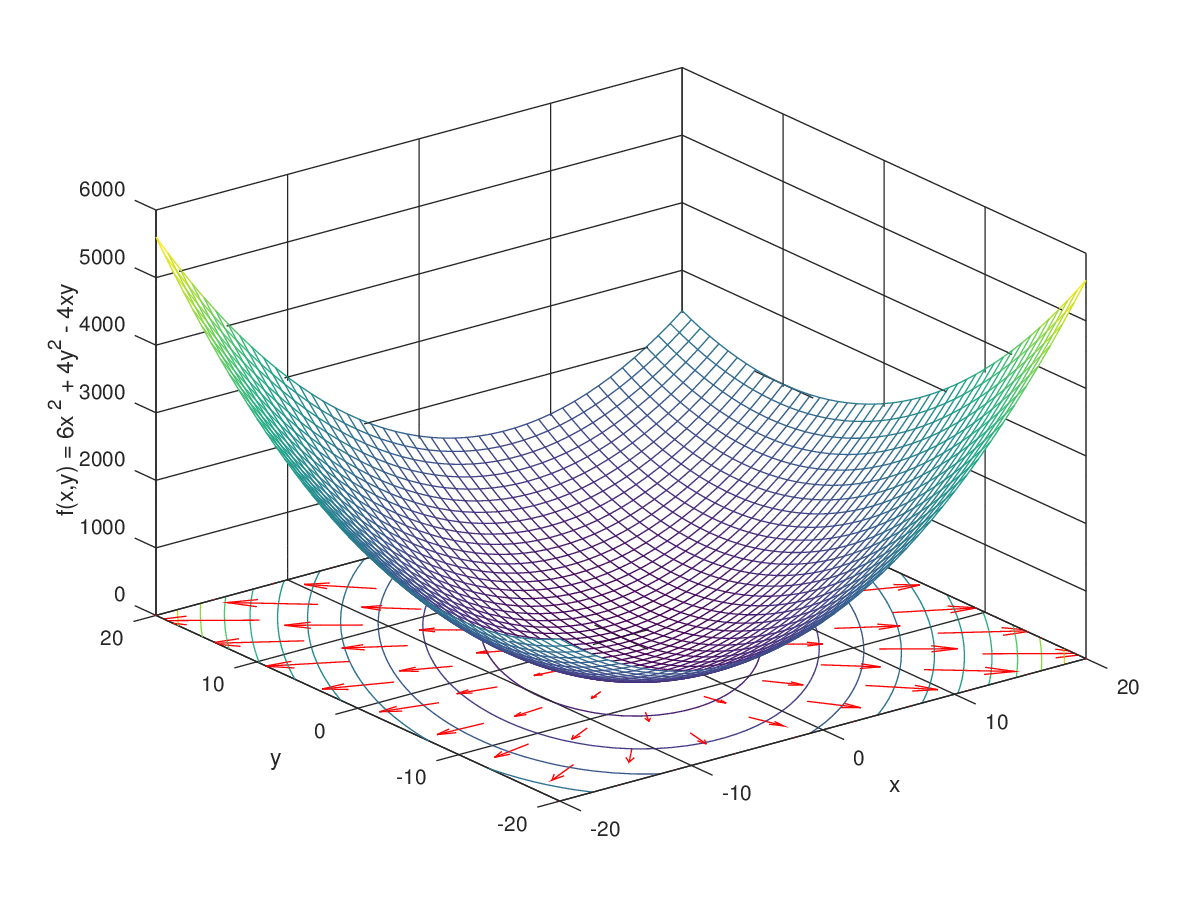
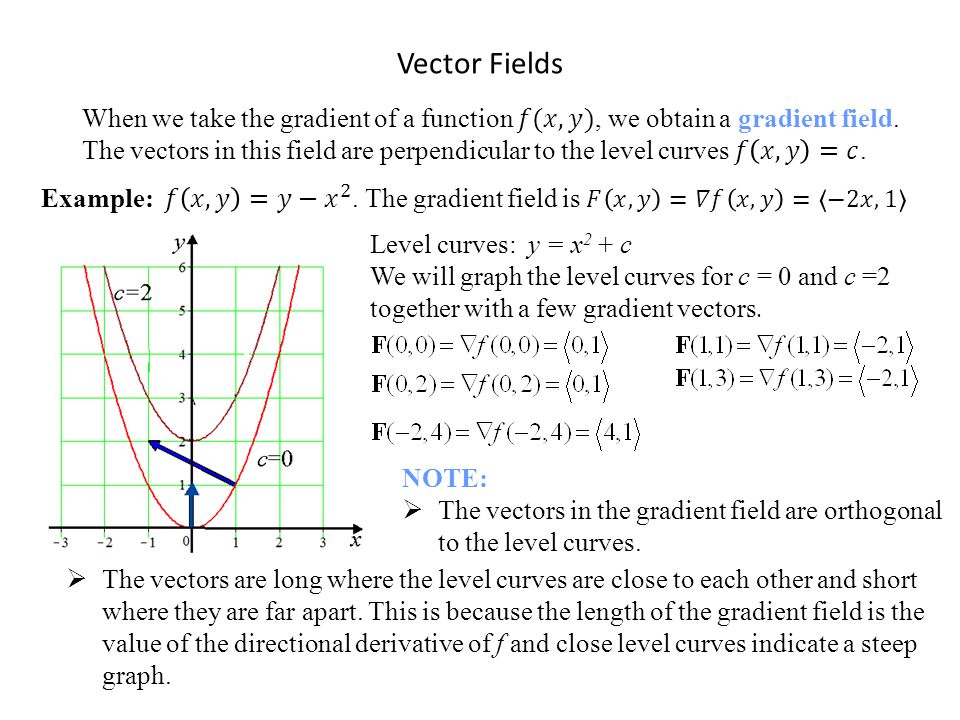
It is also called a conservative vector fieldand is discussed in depth in section 47. The gradient of a function is a vector eld. The gradient of a function fxy in two dimensions is dened as. The gradient or gradient vector field of a scalar function fx1 x2 x3 xn is denoted f or f where nabla denotes the vector differential operator del.

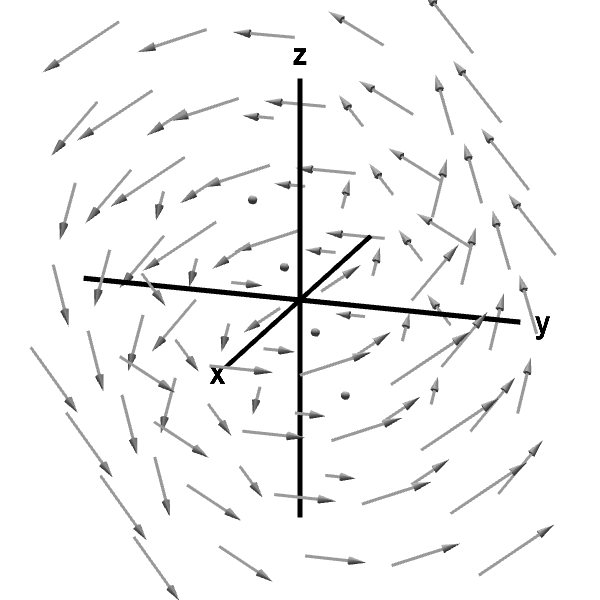
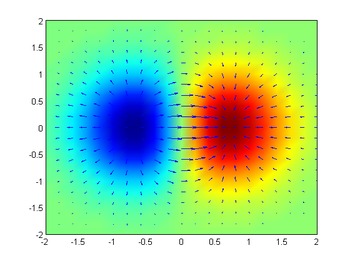
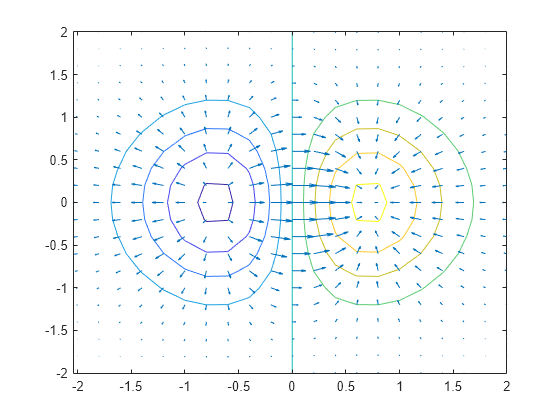
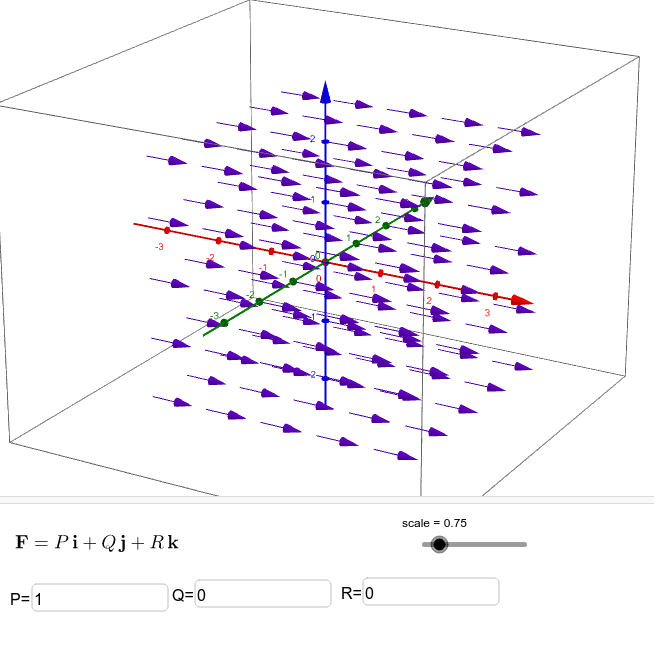
Common examples of vector fields include force fields velocity fields gravitational fields magnetic fields and electric fields. Such a vector eld is called a gradient or conservative vector eld. It is obtained by applying the vector operator to the scalar function fxy. Gradient of element wise vector function combinations.

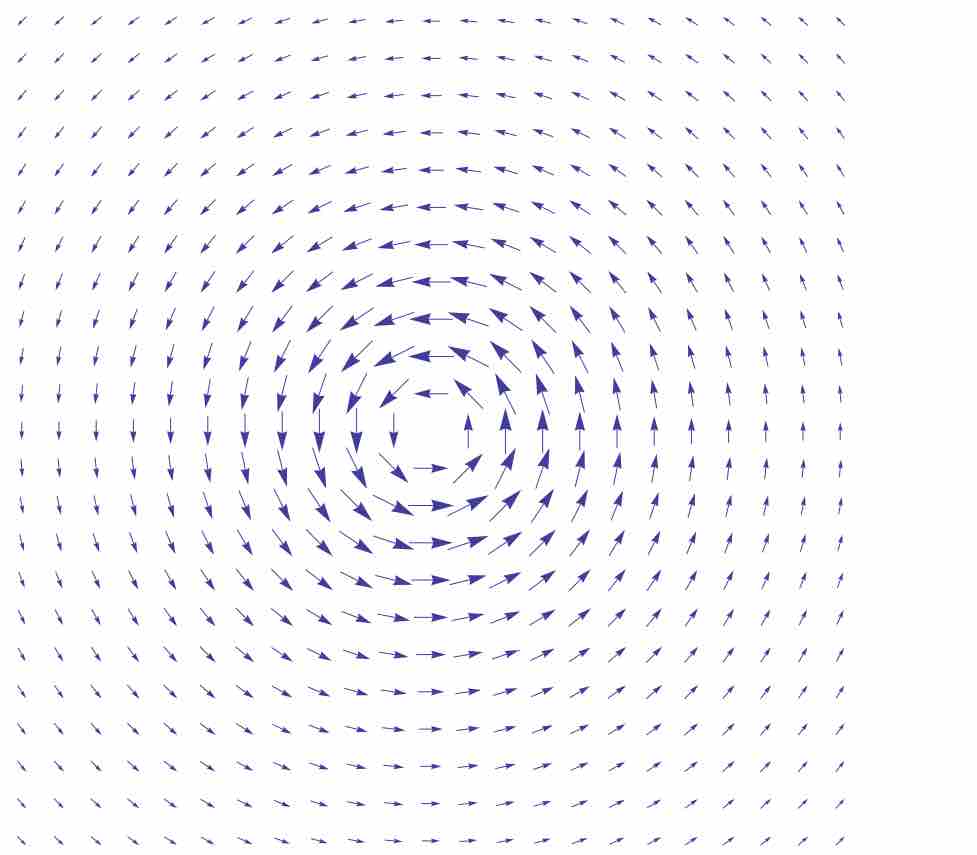
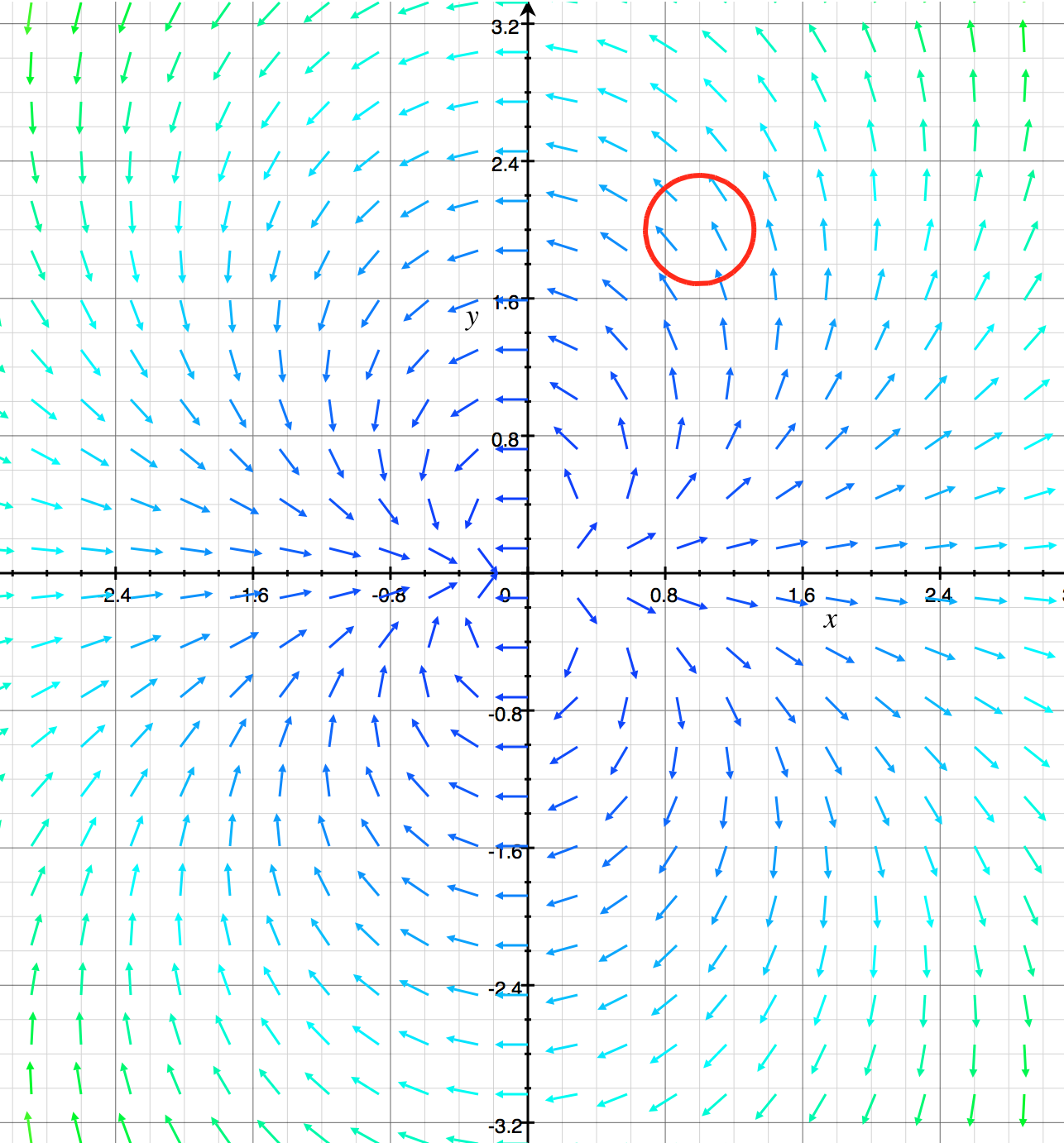
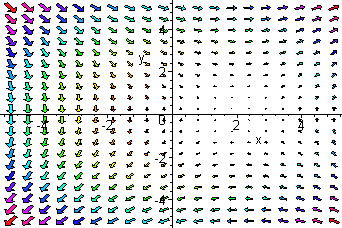
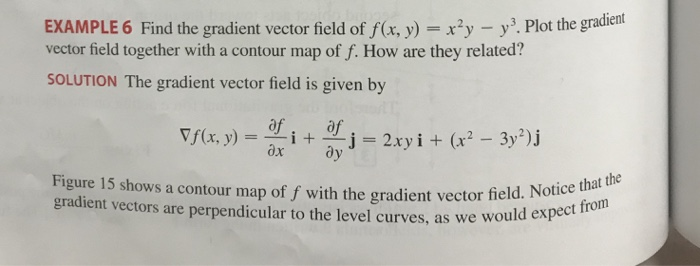
This is called a gradient vector fieldor just gradient field. Vector fields can be used to quantify the amount of work done by a variable force acting on a moving body. If we want to find the gradient at a particular point we just evaluate at that point. Nabla f xyleftlanglefrac partial f partial x xyfrac partial f partial y xyrightrangle f x y.

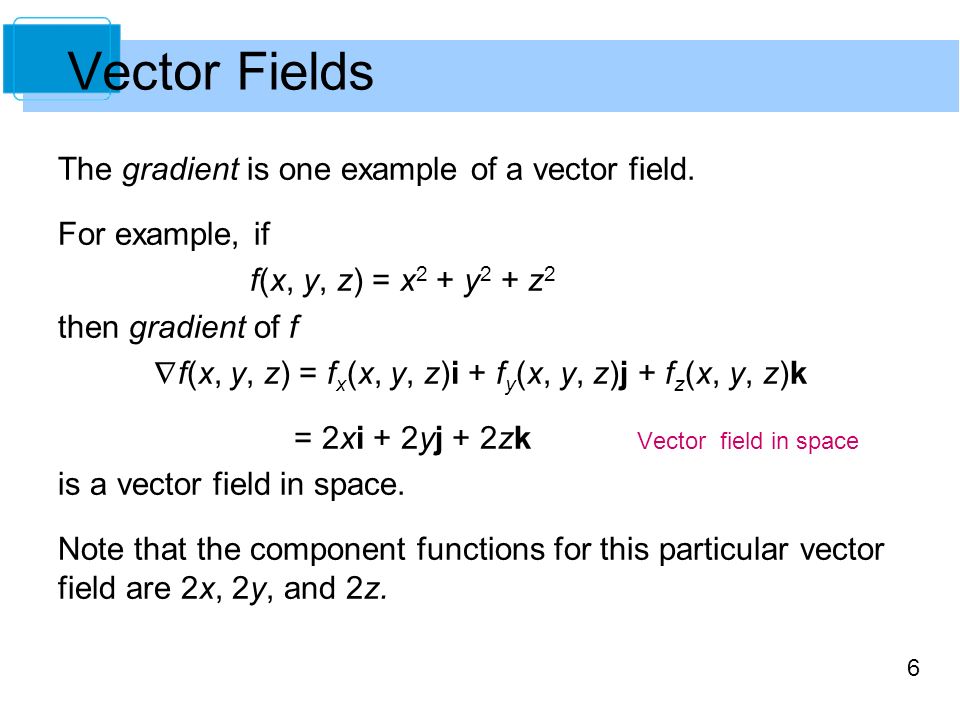
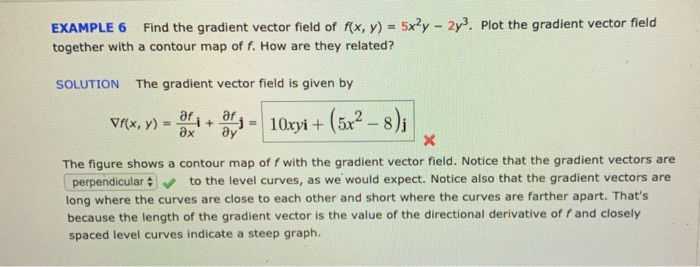
Gradfxy fxy f x i f y j. Points in the direction of greatest increase of a function intuition on why is zero at a local maximum or local minimum because there is no single direction of increase the term gradient is typically used for functions with several inputs and a single output a scalar field. This gives a vector valued function that describes the functions gradient everywhere. In such a case the vector field is written as.

Element wise binary operators are operations such as addition wx or wx which returns a vector of ones and zeros that applies an operator consecutively from the first item of both vectors to get the first item of output then the second item of both vectors to get the second item of outputand so forth. This is a vector field and is often called a gradient vector field.