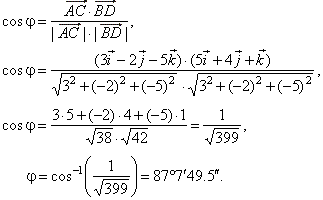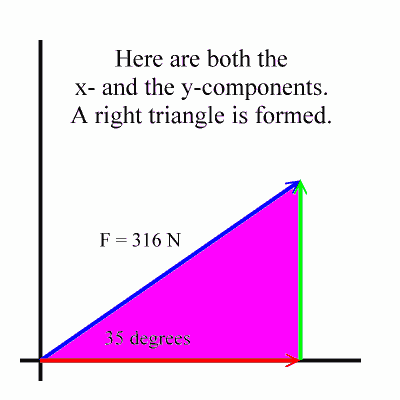How To Find Components Of A Vector

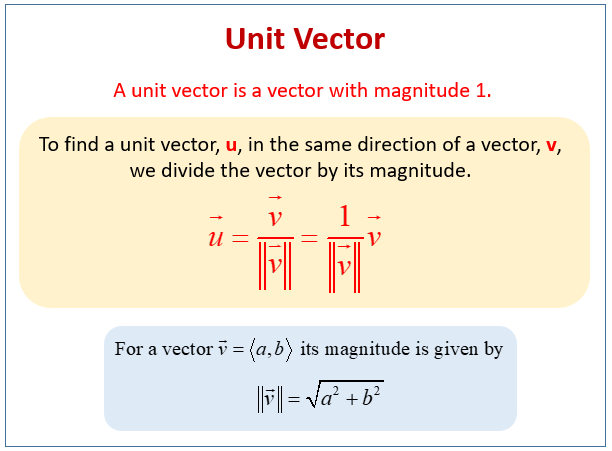
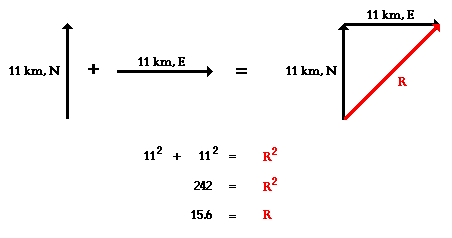
V v x 2 v y 2.

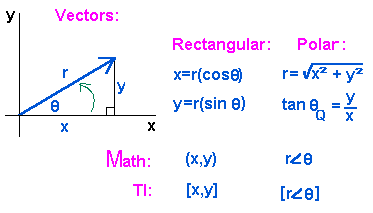
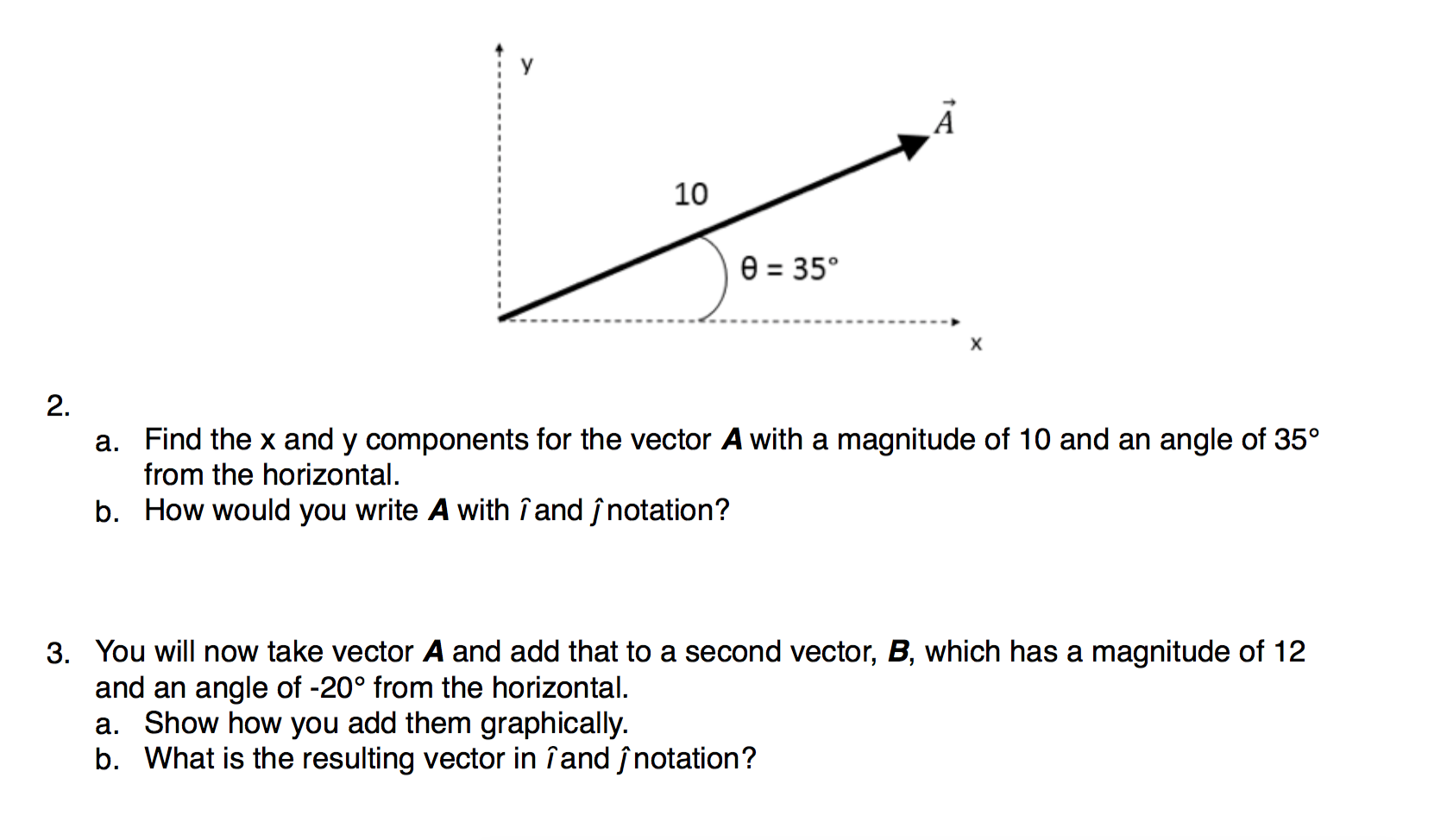
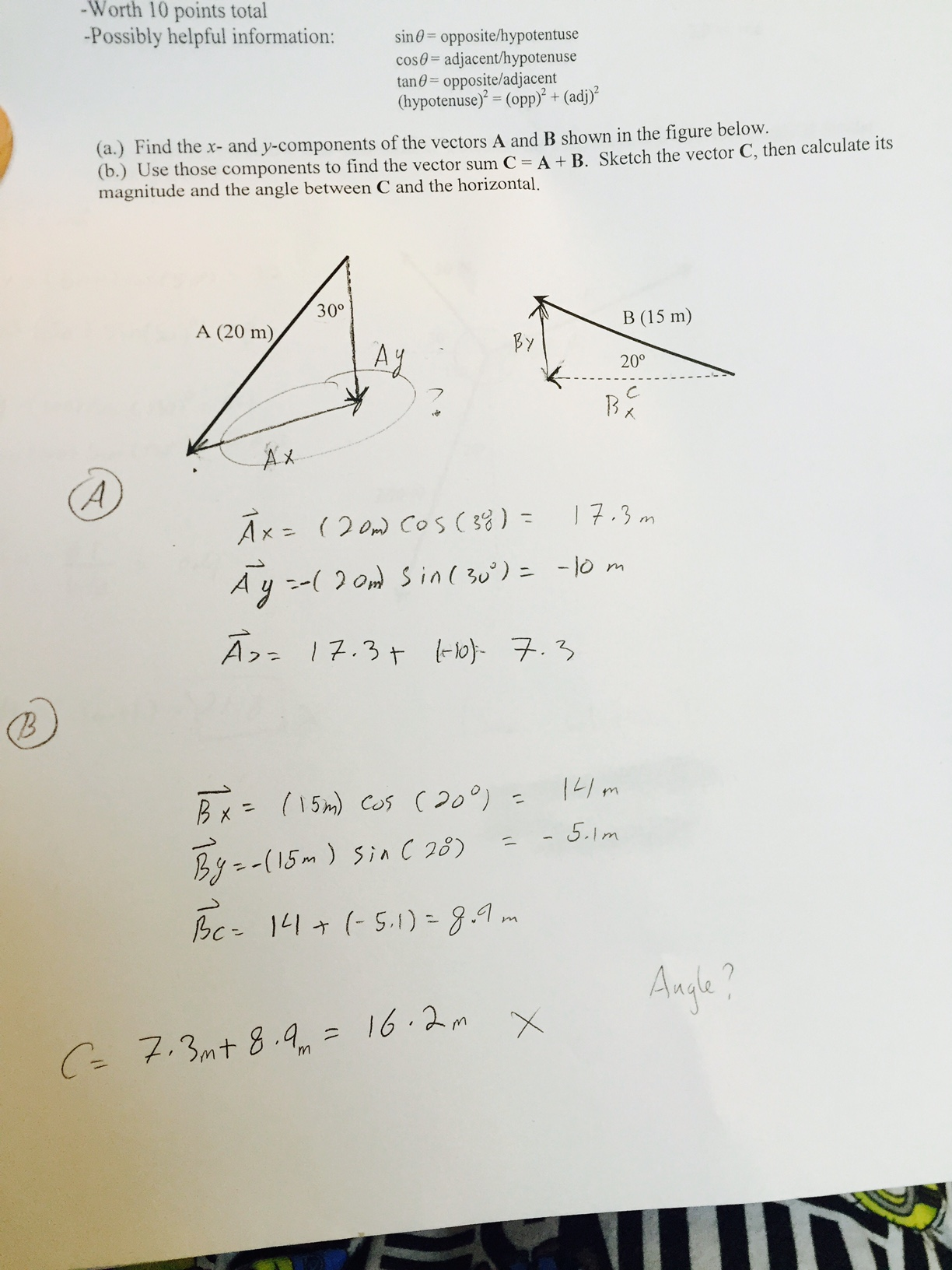
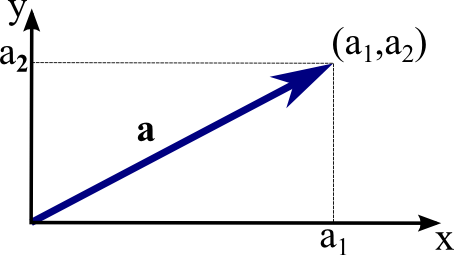
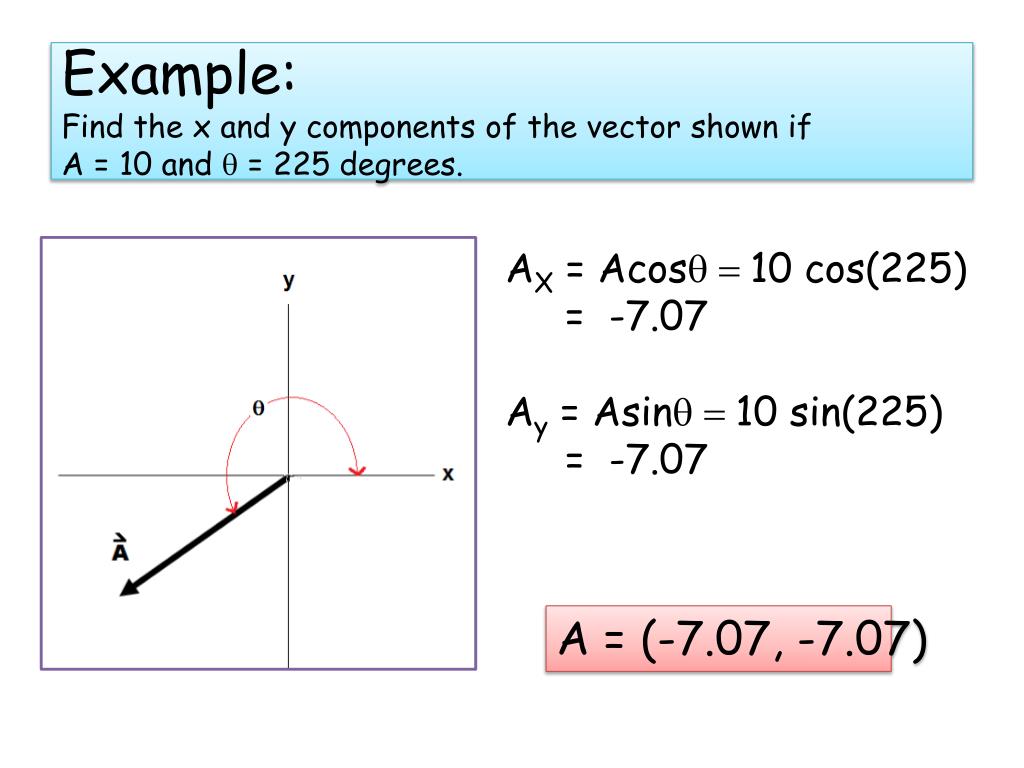
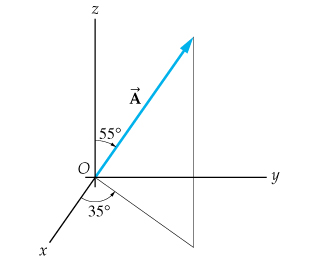
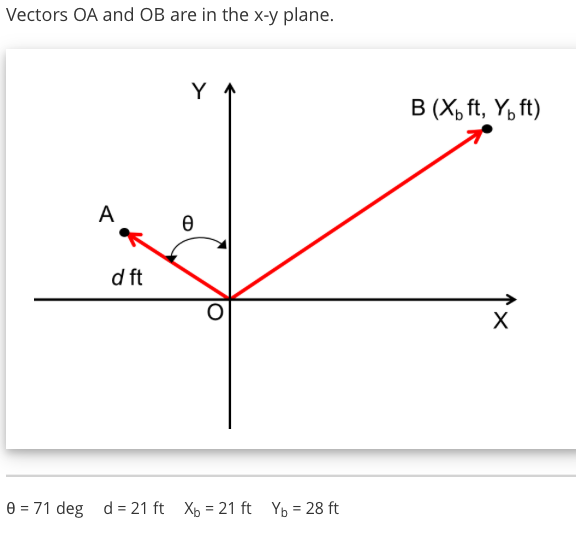
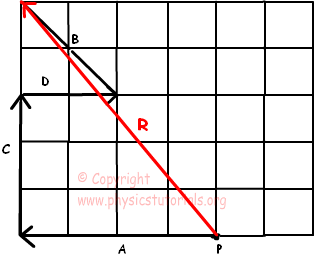
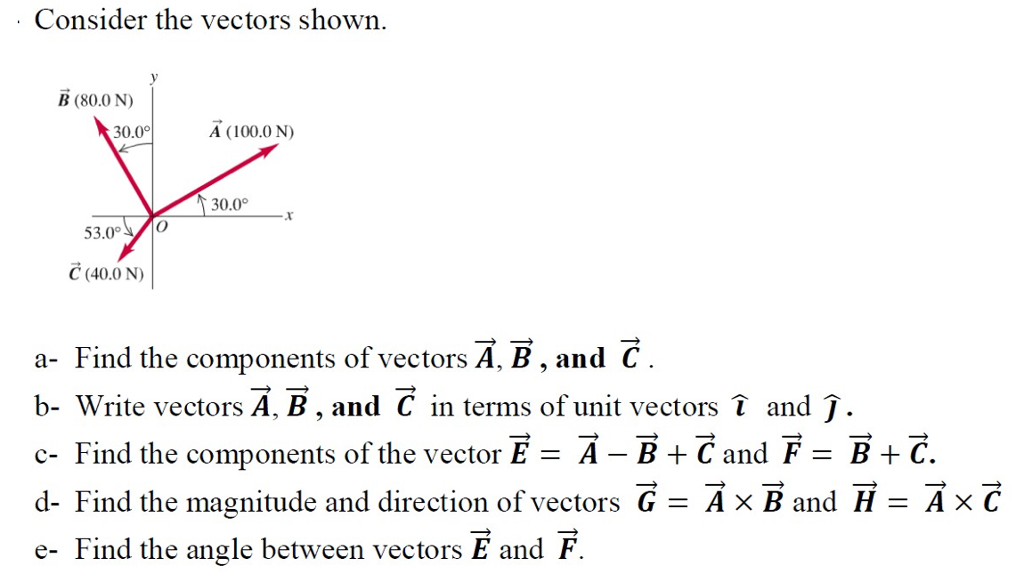
How to find components of a vector. The projections of vector a along the x y and z directions are a x a y and a z respectively. Therefore we can calculate the module of a vector directly if we know the coordinates of the points of its origin and of its end just by replacing in the previous calculation formula of the module the components x and y by the subtraction of the coordinates of the end minus the coordinates of the origin. You have to find the components yourself using a little trigonometry. Here the numbers shown are the magnitudes of the vectors.

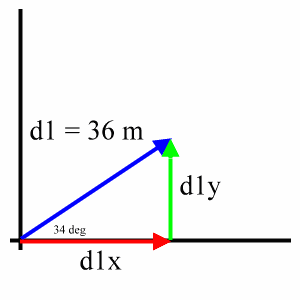
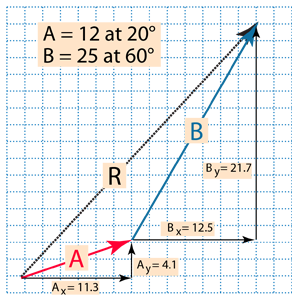
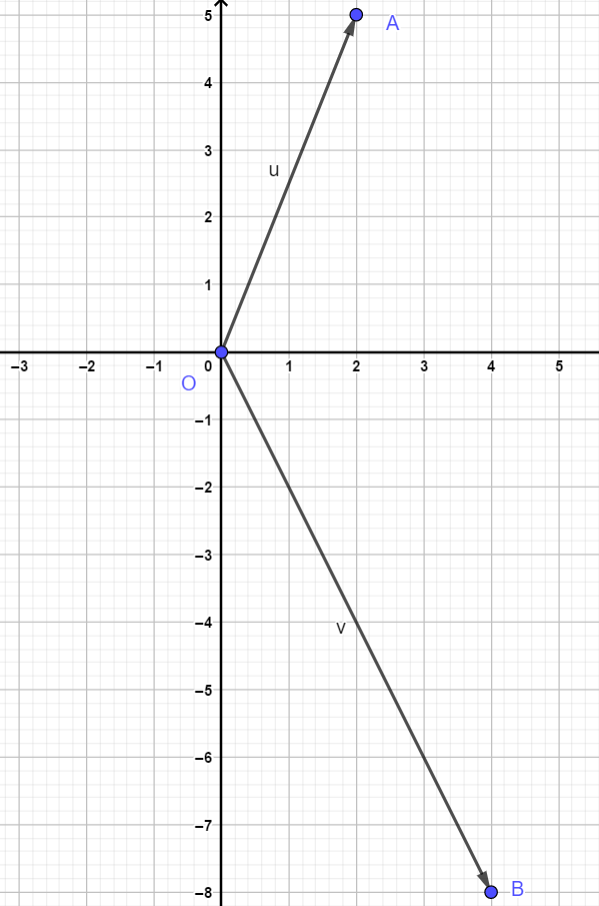
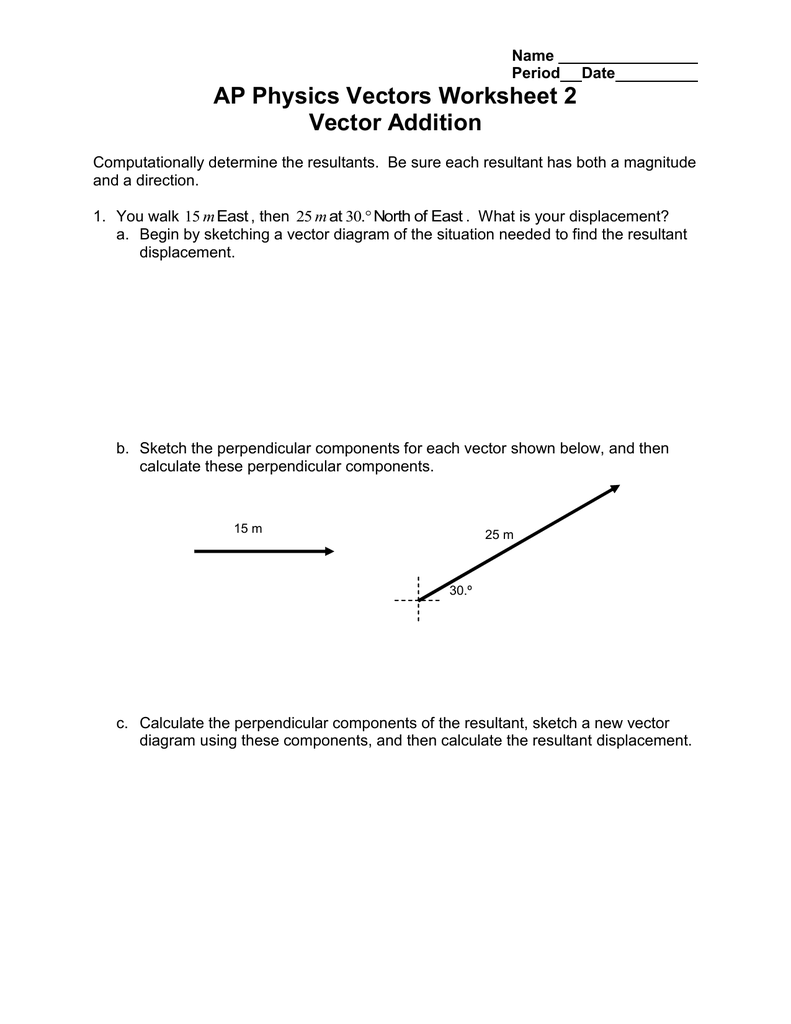
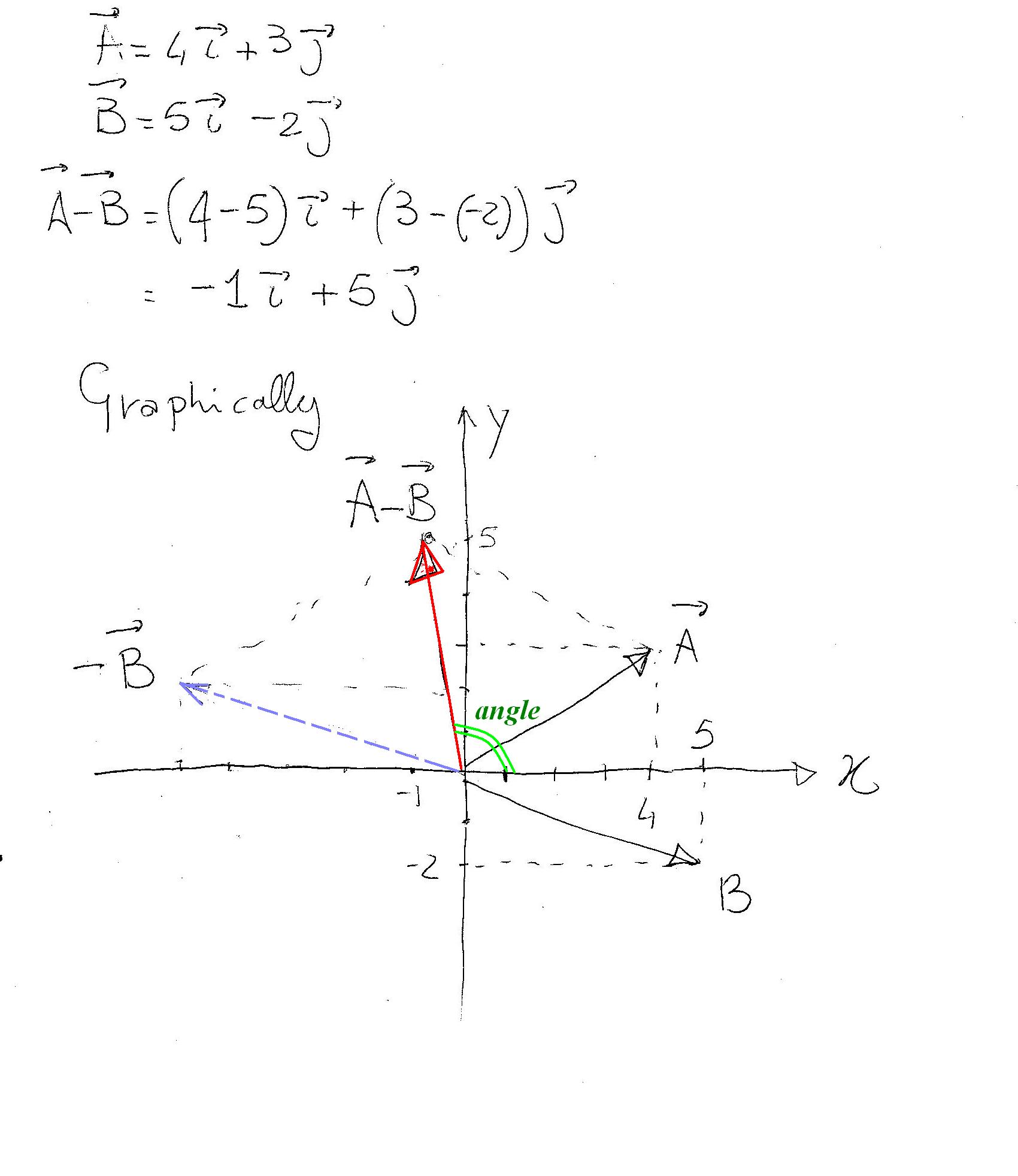
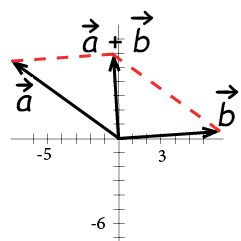
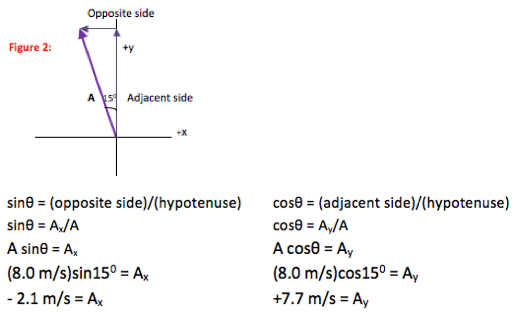
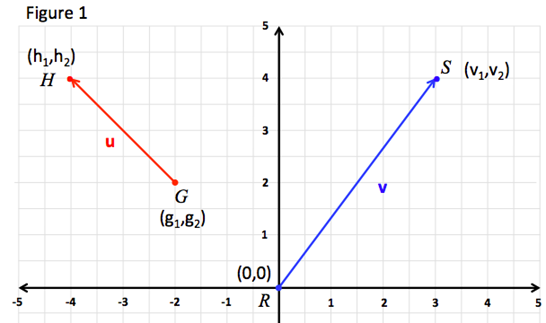
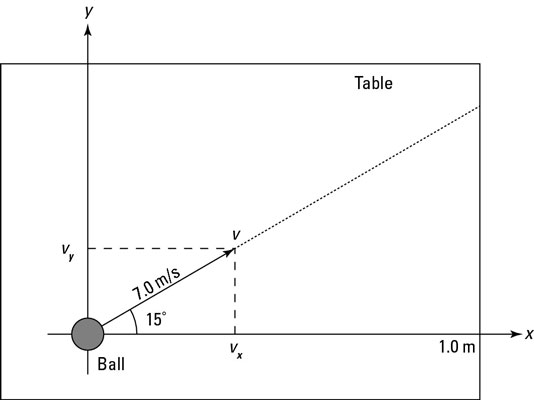
Suppose you know that a ball is rolling on a flat table at 15 degrees from a direction parallel to the bottom edge at a speed of 70 meterssecond. The components of a vector depict the influence of that vector in a given direction. It can be represented as v v x v y where v is the vector. Let u 21 3j and v 4i7.

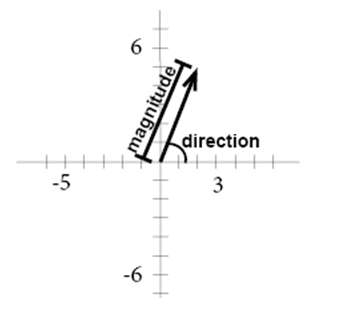
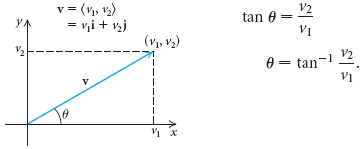
Typically a physics problem gives you an angle and a magnitude to define a vector. Each part of a two dimensional vector is known as a component. To find direction of the vector solve tan o v y v x for. Get 11 help now from expert calculus tutors solve it with our calculus problem solver and calculator.

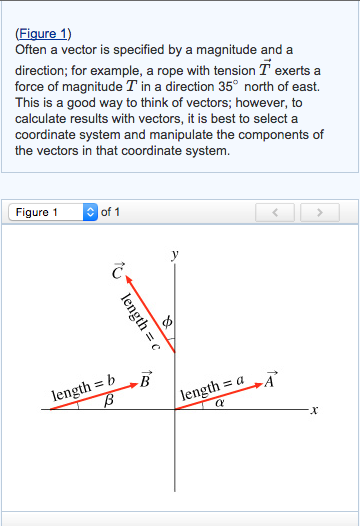
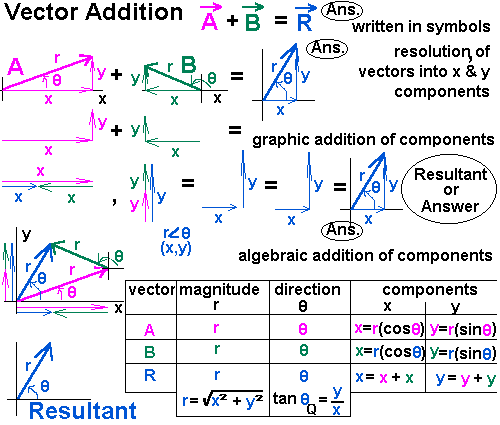
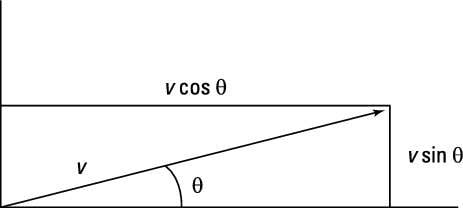
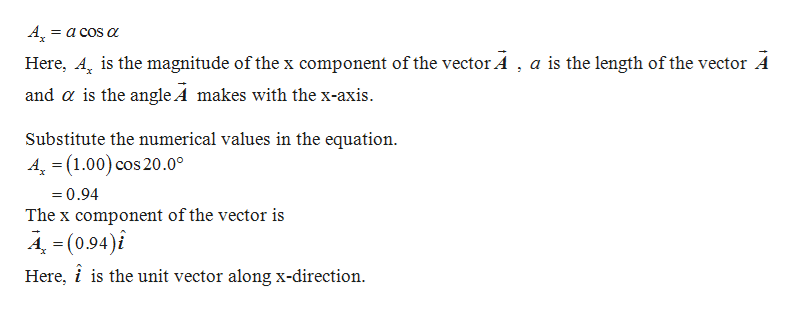
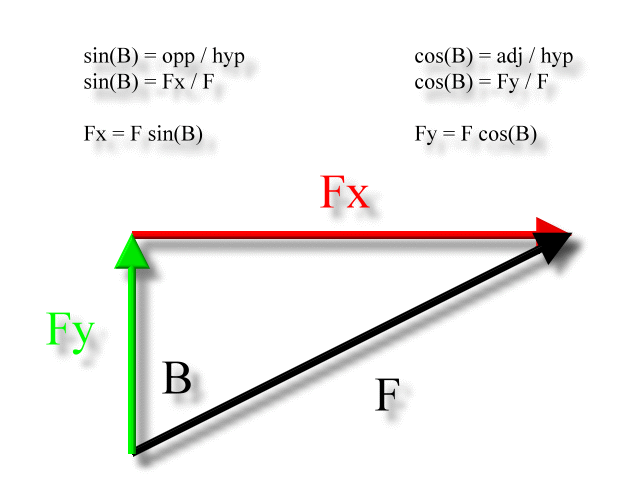
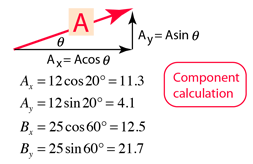
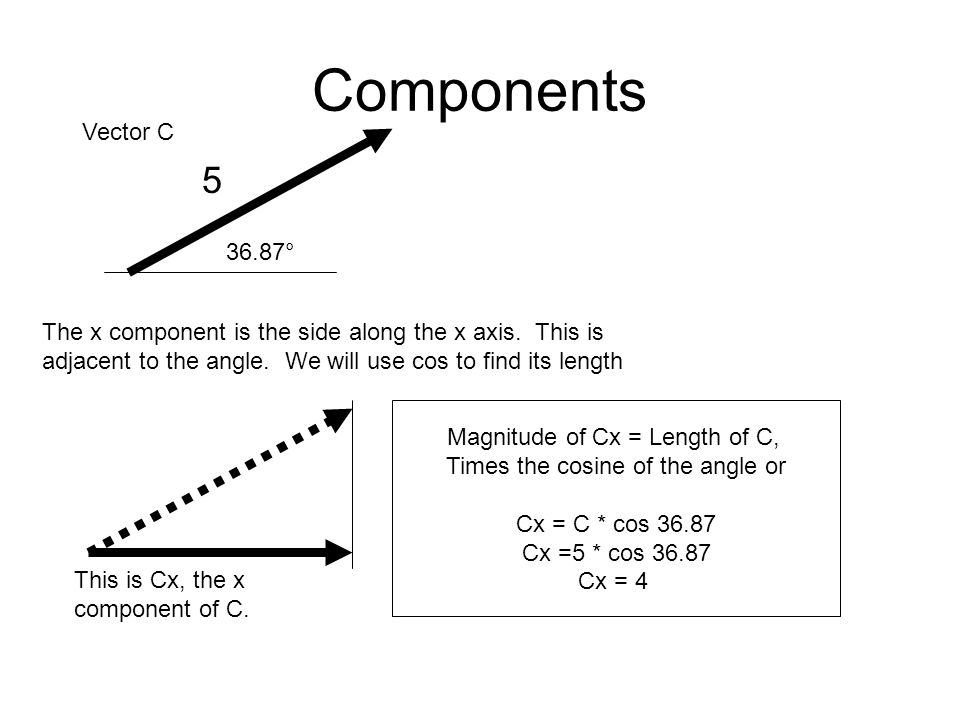
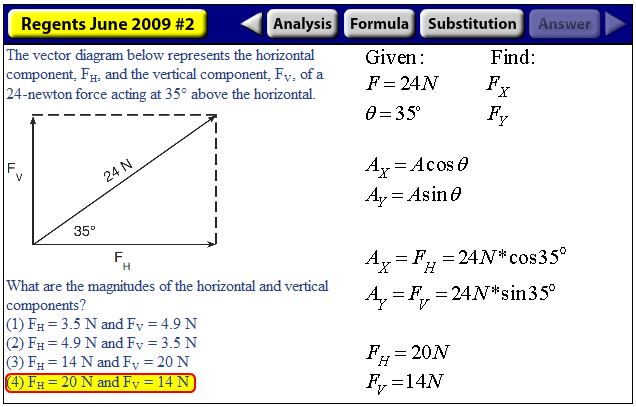
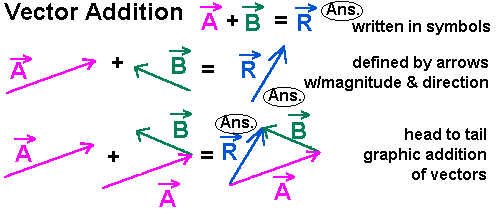
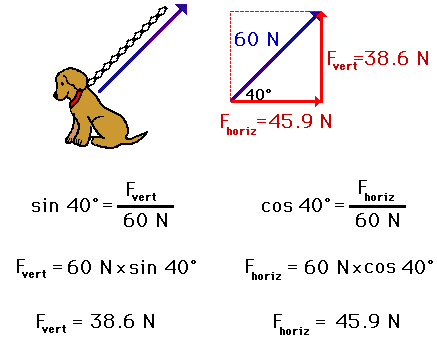
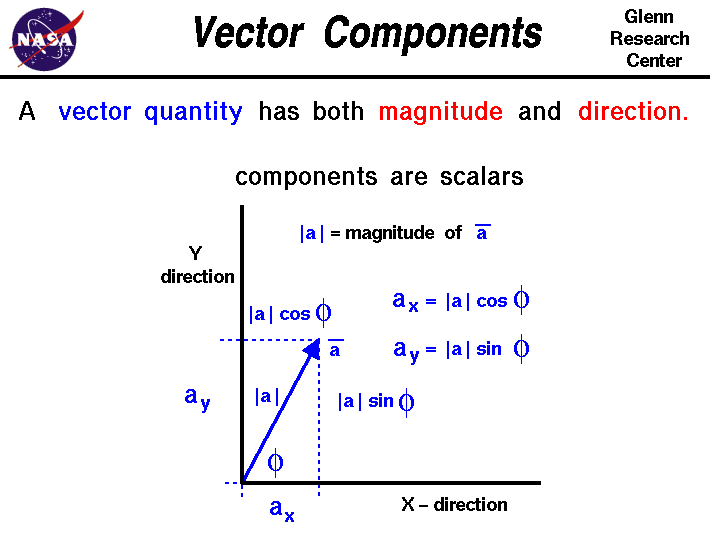
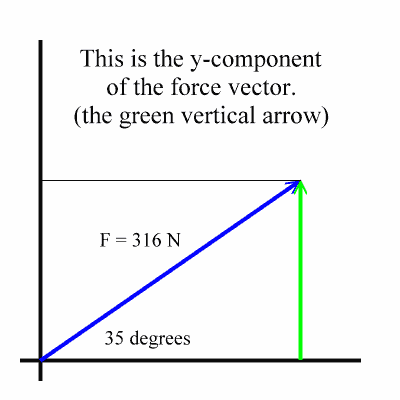
Components of a vector. In the following diagram a is the angle that the two dimensional force vector makes with the x axis. If you know a vectors vertical and horizontal components finding the vectors magnitude isnt so hard because you just need to find the hypotenuse of a right triangle. Let the angle between the vector and its x component be o.

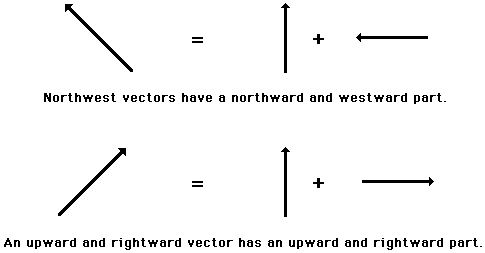
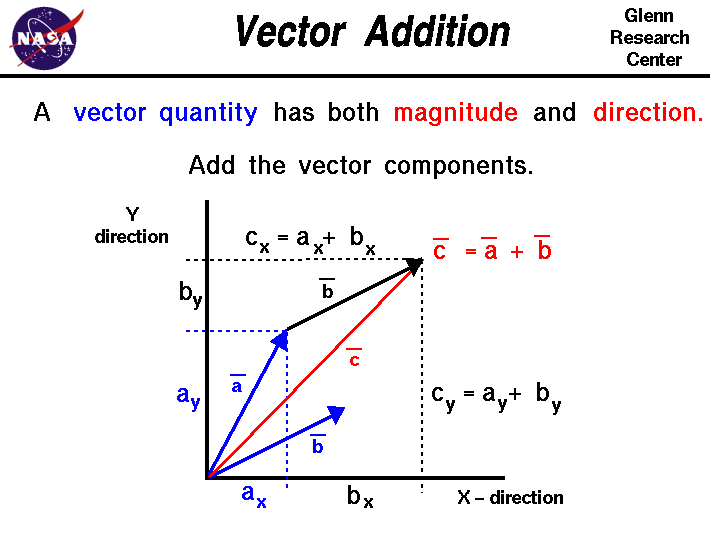
That is it can be thought of as having two parts. Any vector directed in two dimensions can be thought of as having an influence in two different directions. Plugging in the numbers gives you. The vector and its components form a right angled triangle as shown below.

If we know the size of the two dimensional force vector the black one in the above diagram and the angle it makes with the x axis then we can use right triangle trigonometry to find the values for the components. These are the parts of vectors generated along the axes. A b 2v d find the dot product of vector u and v. Ex 102 5 introductionfind the scalar and vector components of the vector with initial point 2 1 and terminal point 5 7let vector 2 5 4 thenscalar components 2 5 and 4vector components 2 5 and 4 ex 102 5 find the scalar and vector c.

Rectangular component of a vector. The components of a vector in two dimension coordinate system are usually considered to be x component and y component. Magnitude of a vector. Get more help from chegg.

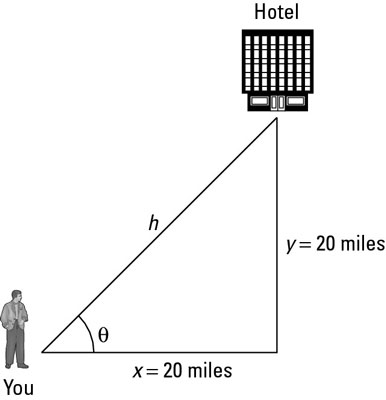
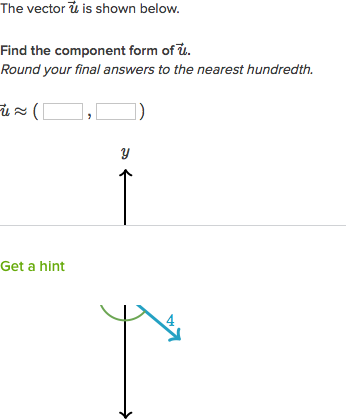
Find the components of a vector with an initial position of 3 1 and a terminal position of 4 5. You can use the pythagorean theorem x2 y2 h2 solved for h.