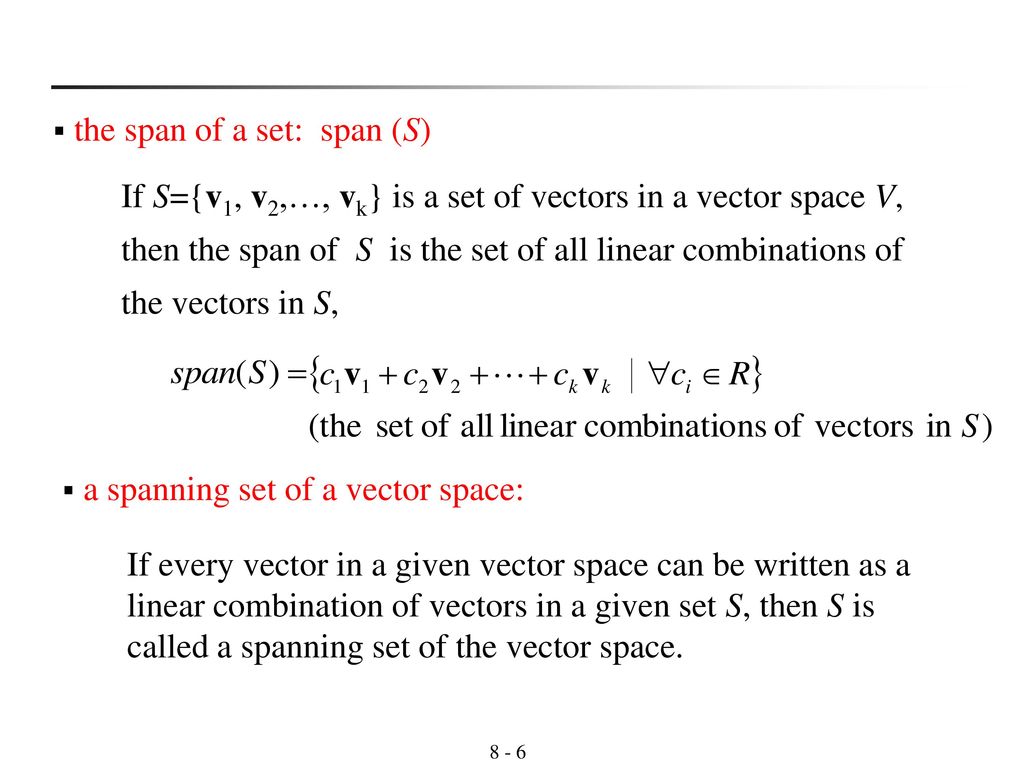
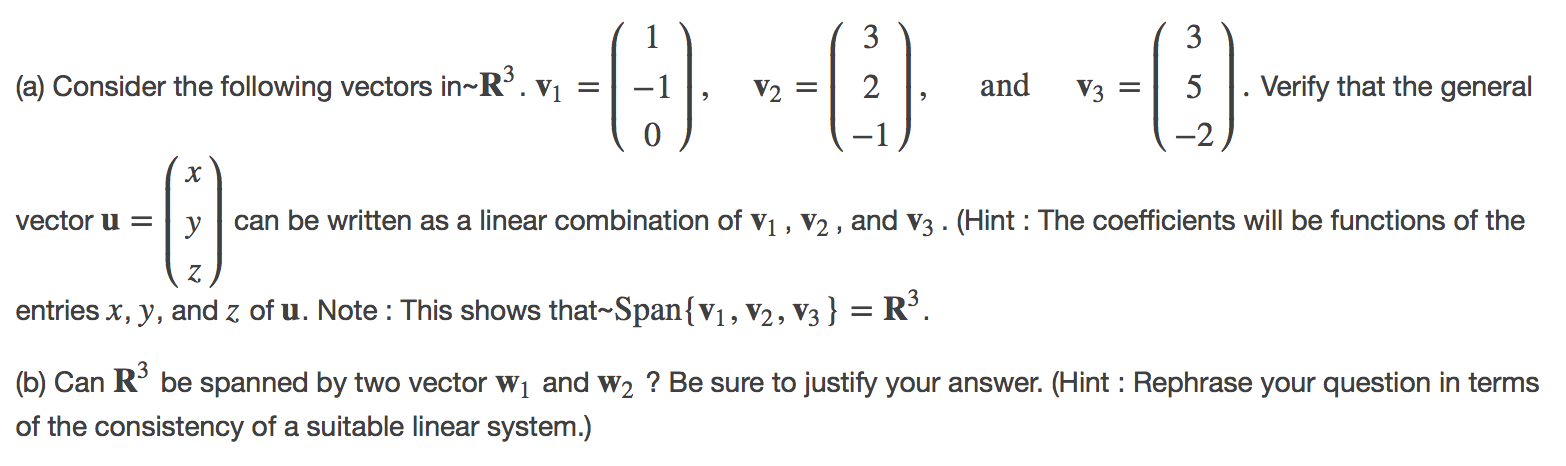
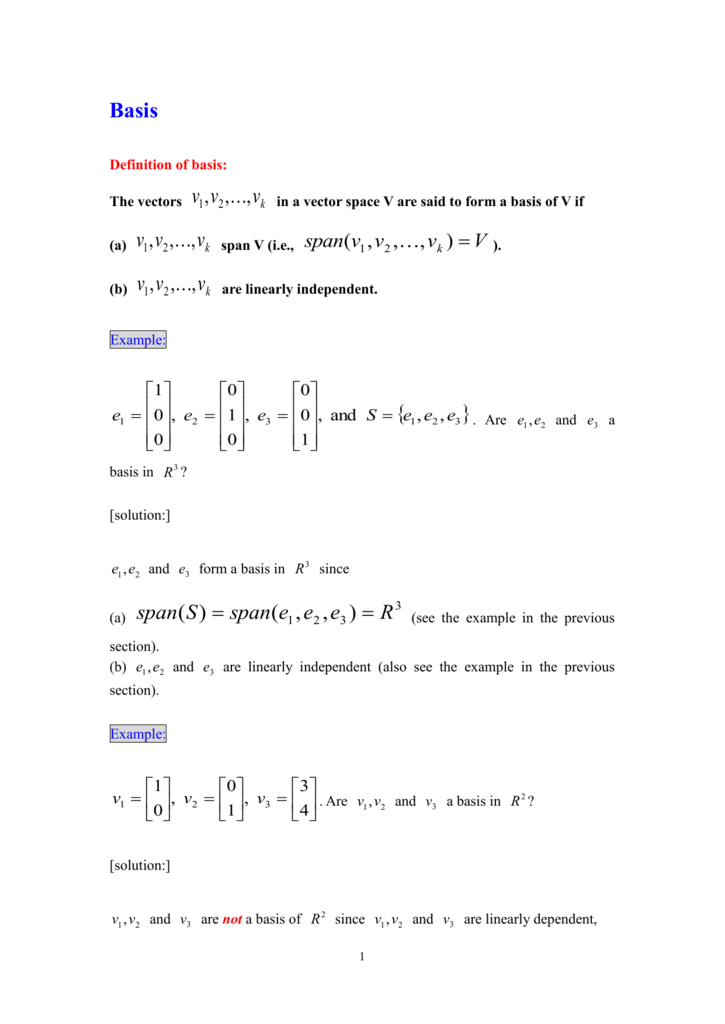
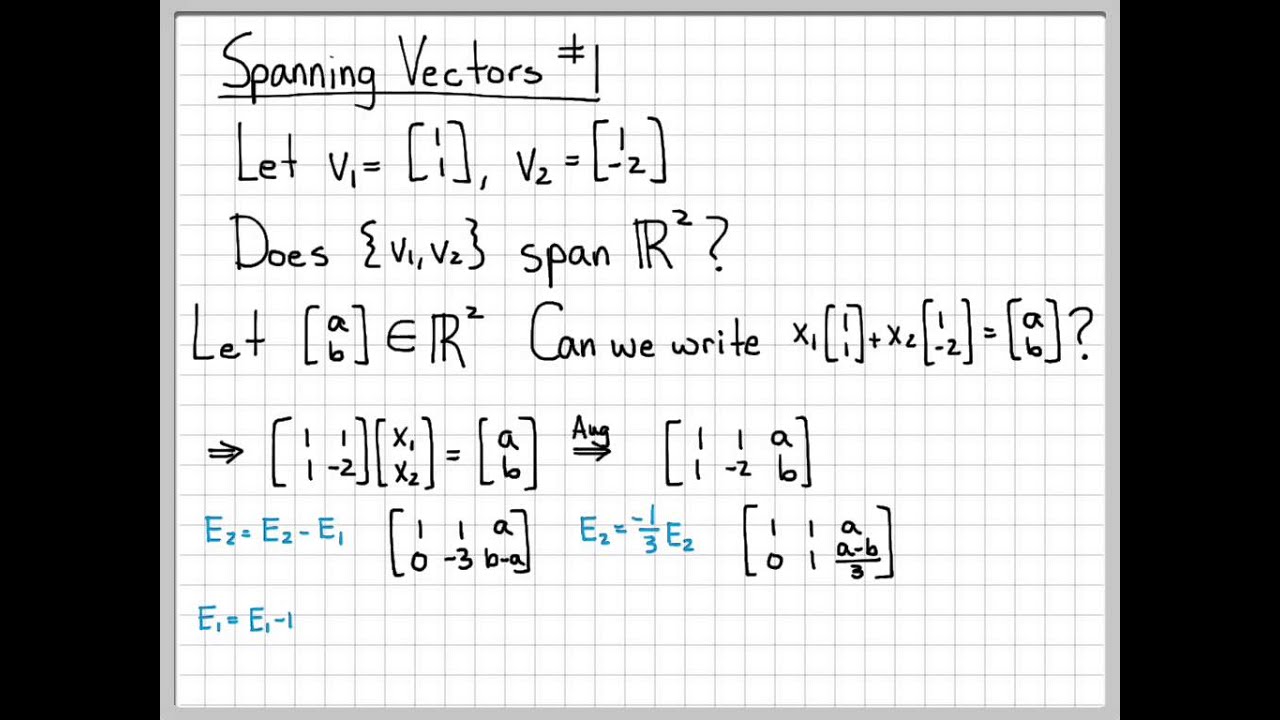
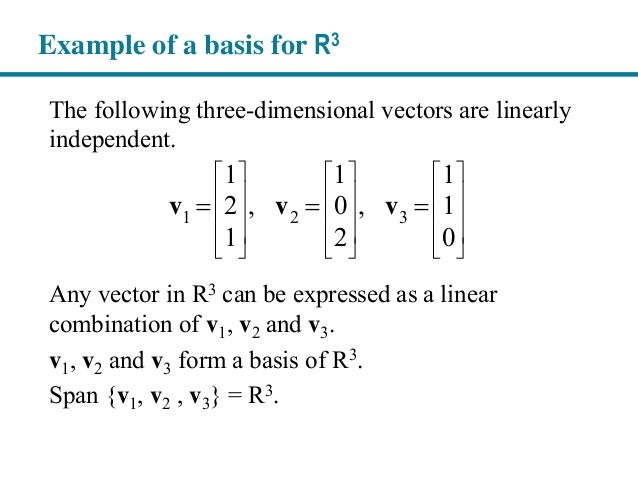
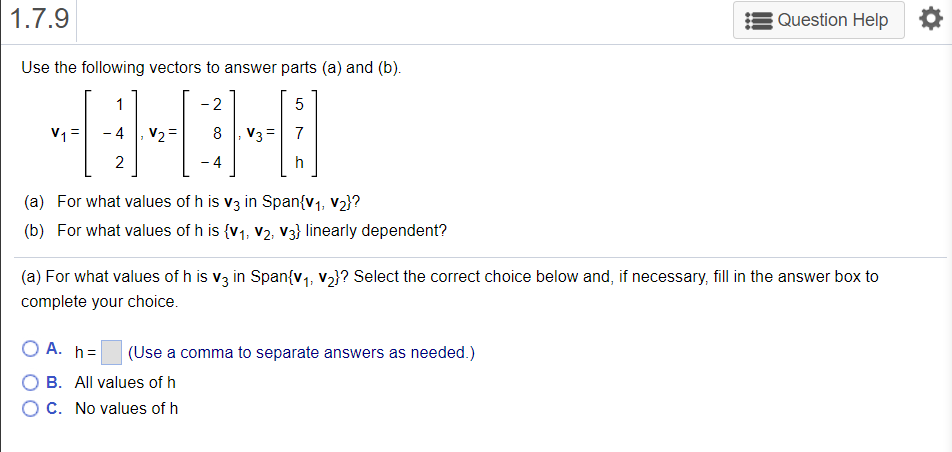
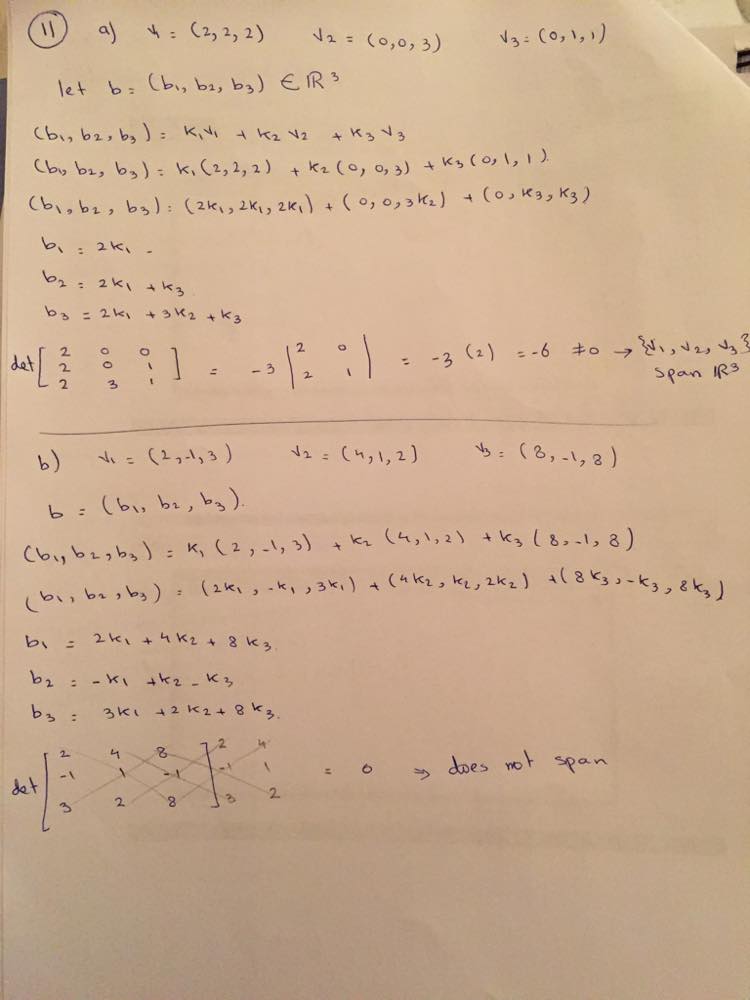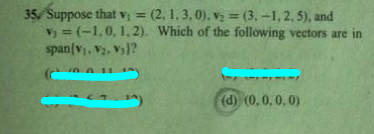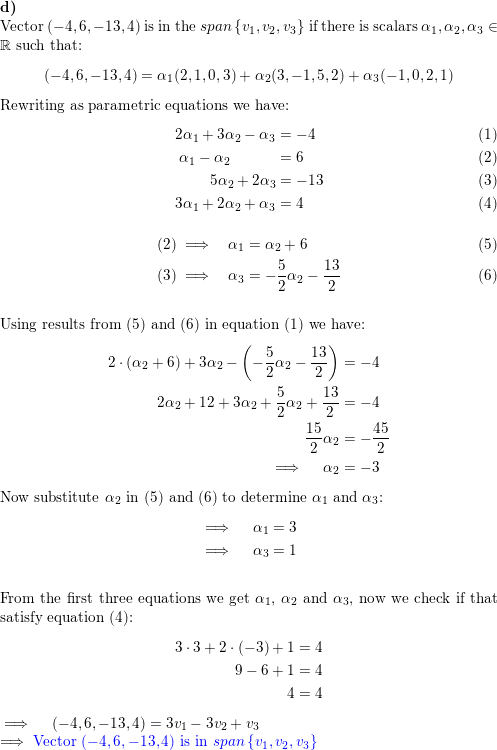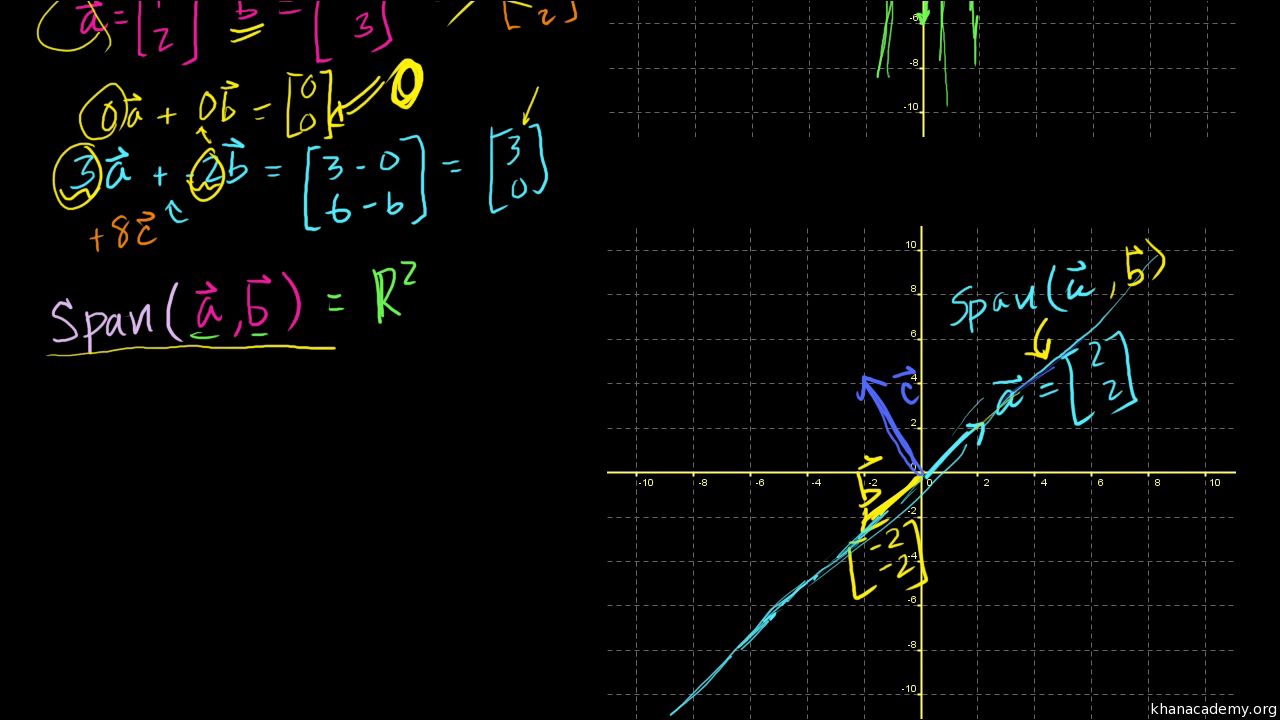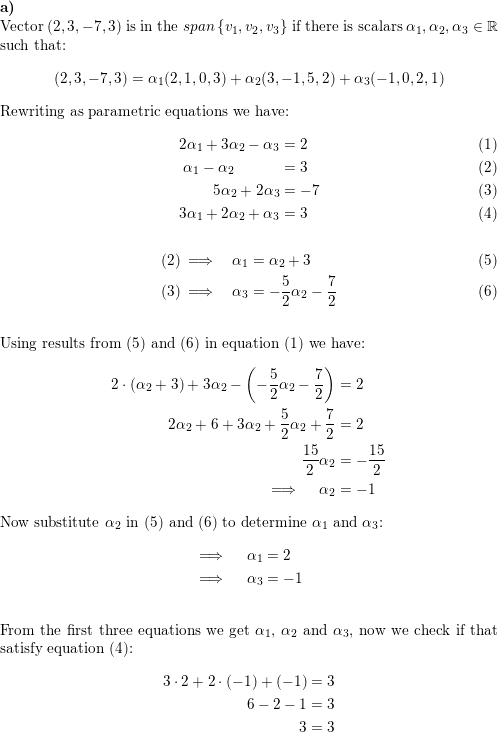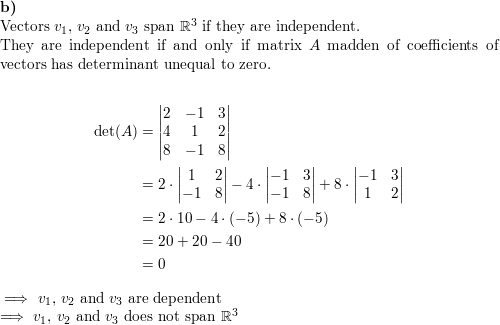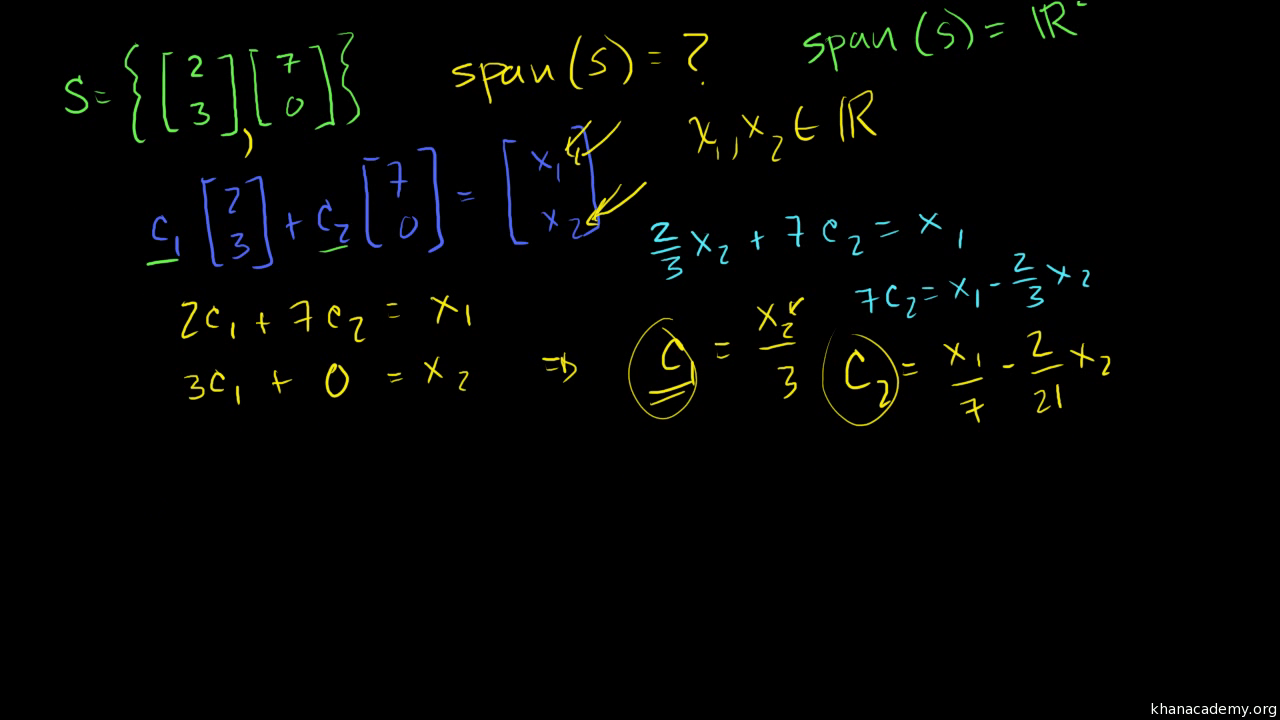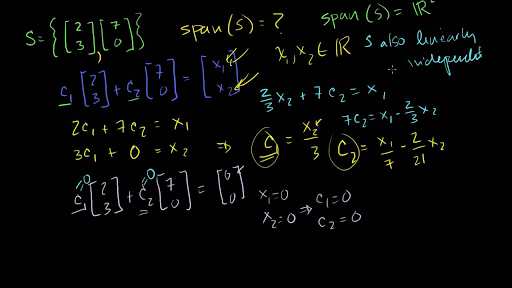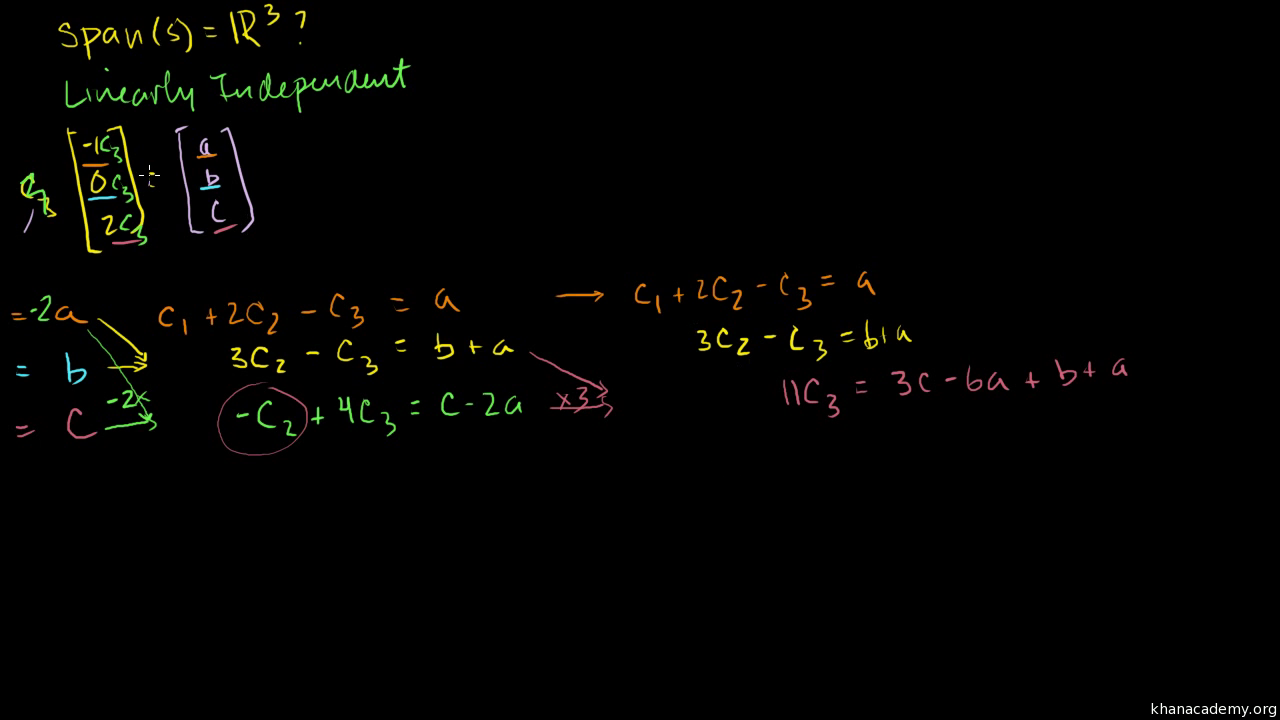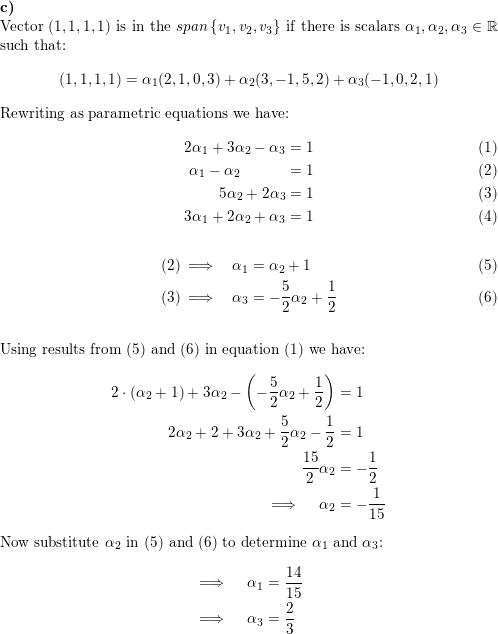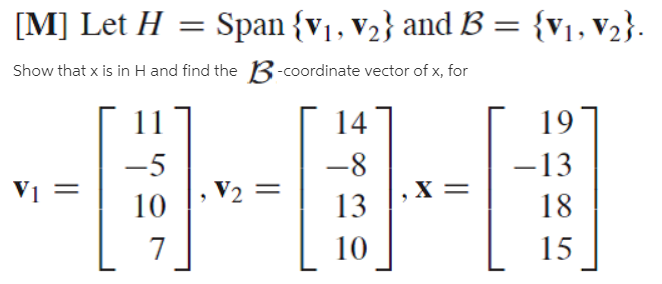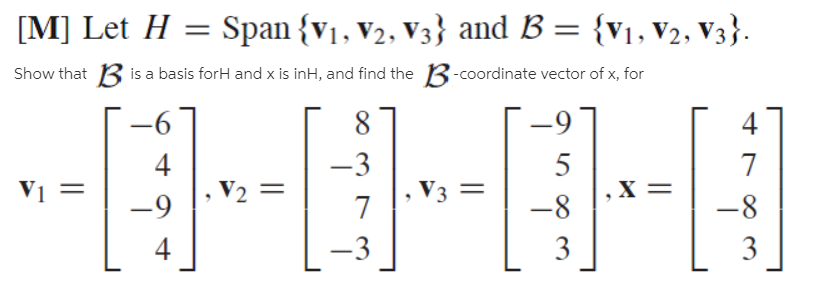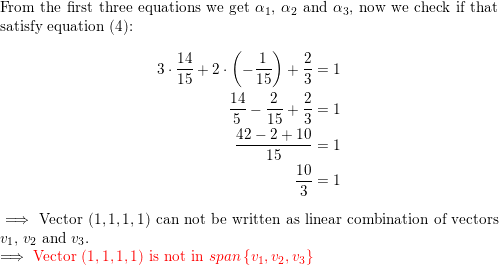Which Of The Following Vectors Are In Span V1 V2

We will give two solutions.

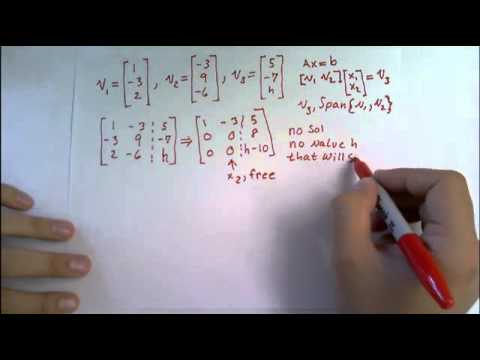
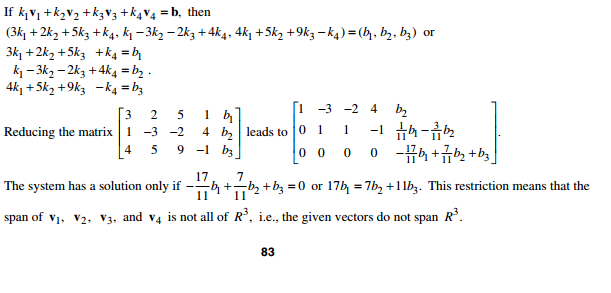
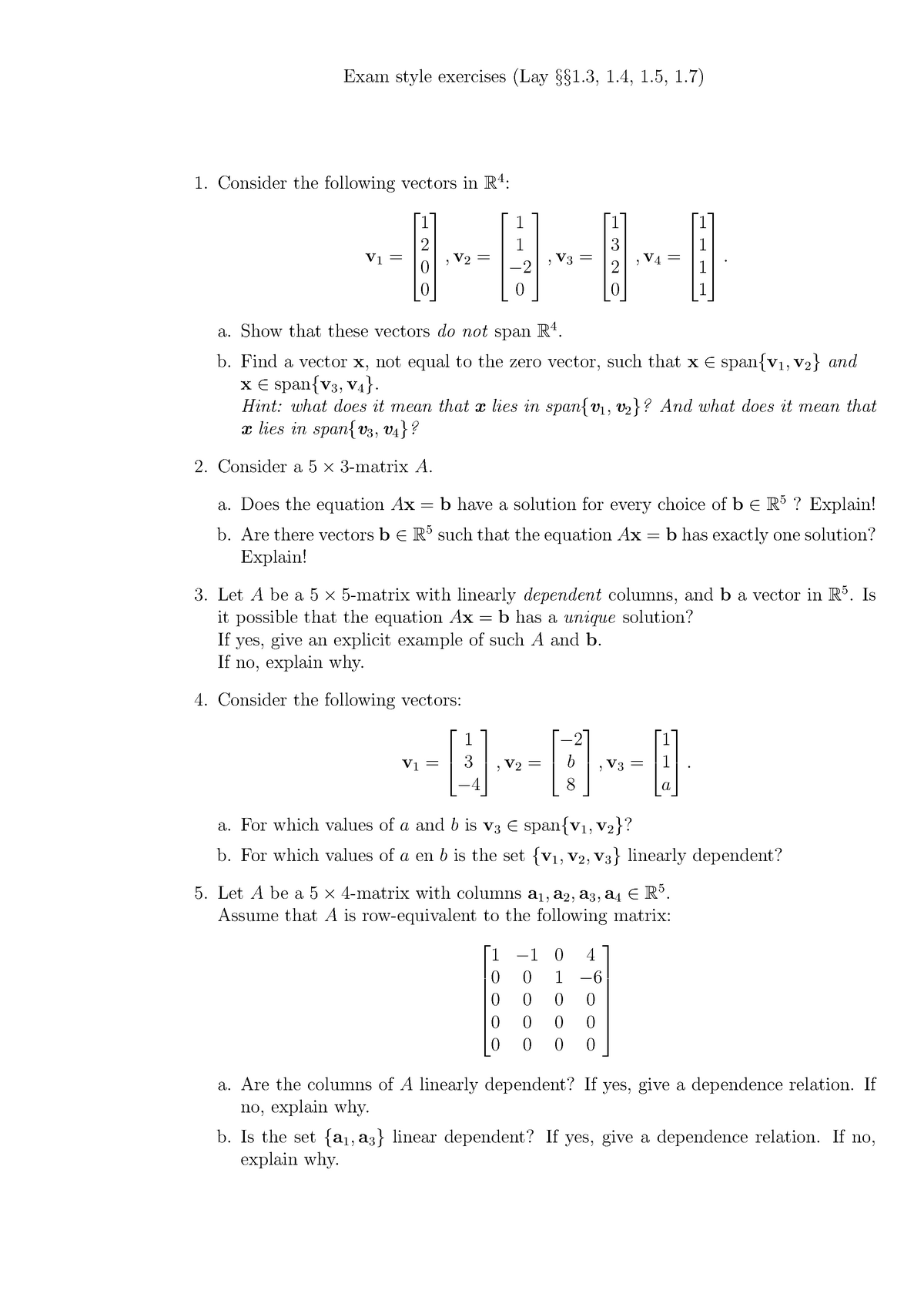
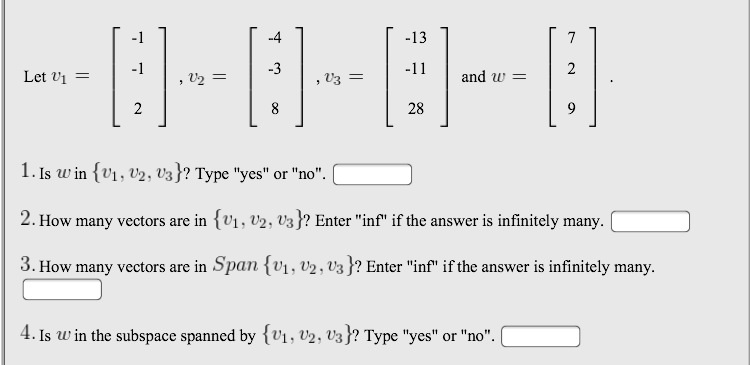
Which of the following vectors are in span v1 v2. Problem 20e from chapter 13. Let h span v1 v2 v3 v4. Do not make a sketch is broken down into a number of easy to follow steps and 35 words. Our aim is to solve the linear system ax v where a 2 4 1 2 4 1 1 3 4 3 5 3 5and x 2 4 c 1 c 2 c 3 3 5.

Which of the following vectors are in span v1v2v3. If a vector is in the indicated span then write it as a linear combination of the vectors above. Span then show that it cannot be written as a linear combination of the vectors above. Linear algebra and its applications 5th edition edit edition.

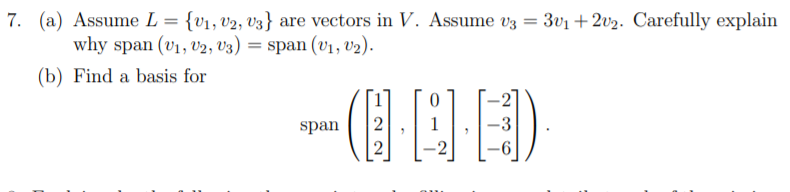
Find a basis for the subspace v. For each of the following sets of vectors determine whether h is a line plane or r3. If you take the span of two vectors in r 2 the result is usually the entire plane r. For each vector show the weights on v1 and v2 used to generate the vector and list the three entries of the vector.

For an arbitrary v 2r3. If a vector is not in the indicated. Vector list sketch make show. Understanding linear combinations and spans of vectors.

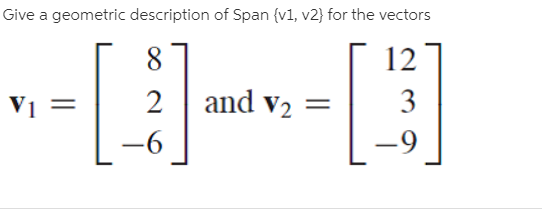
Understanding linear combinations and spans of vectors. Thus the span of these three vectors is a plane. Give a geometric description of span v1 v2 for the vectors. This has a solution only when 7x11y z 0.

Let v be a subspace in r4 spanned by five vectors. If youre seeing this message it means were having trouble loading external resources on our website. If v xyz reduce the augmented matrix to 2 4 1 2 4 x 0 1 1 x y 0 0 0 7x11y z 3 5. If you take the span of two vectors in r3 the result is usually a plane through the origin in 3 dimensional space.